User:Dmitrii Kouznetsov/Analytic Tetration: Difference between revisions

From Citizendium
Jump to navigation Jump to search
imported>Dmitrii Kouznetsov
m (→‎Quick start: misprint)
m (Text replacement - "George Orwell" to "George Orwell")
 
(48 intermediate revisions by 4 users not shown)
Line 1: Line 1:
[[Image:AnalyticTetrtionBase2figure0.jpg|200px|right|thumb|Fig.0. Graphic of analytic tetration <math>f={\rm sex}_2(x)=\exp_2^x(1)</math> at versus <math>x</math>.]]
{{AccountNotLive}}
{{Image|AnalyticTetrtionBase2figure0.jpg|right|200px|Fig.0. Graphic of analytic tetration <math>f=F_2(x)=\exp_2^x(1)</math> versus <math>x</math>.}}
<!--
<!--
[[Image:ExampleEquationLog01.png|300px|right|thumb|FIg.1. Example of graphic solution of equation <math>L=\log_b(L)</math> for
{{Image|ExampleEquationLog01.png|right|300px|FIg.1. Example of graphic solution of equation <math>L=\log_b(L)</math> for
<math>b=\sqrt{2}</math> (two real solutions, <math>L=2</math> and <math>L=4</math>),
<math>b=\sqrt{2}</math> (two real solutions, <math>L=2</math> and <math>L=4</math>),
<math>b=\exp(1/\rm e)</math> (one real solution <math>L=\rm e</math>)
<math>b=\exp(1/\rm e)</math> (one real solution <math>L=\rm e</math>)
<math>b=2</math> (no real solutions).]]!-->
<math>b=2</math> (no real solutions).}}!-->


{{under construction}}
In this page, I collect pieces and figures, which can be used to build-up [[tetration]].
==Abstract==
==Abstract==


Line 18: Line 19:
Especially I invite  
Especially I invite  
*Arthur Knoebel
*Arthur Knoebel
*Henryk Trappman
*Henryk Trappmann
*Andrew Robbins
*Andrew Robbins
to edit this file.<!-- (they already have found several misprints in my previous postings.)!-->
to edit this file.<!-- (they already have found several misprints in my previous postings.)!-->
Line 34: Line 35:
Roughly, super-exponential  
Roughly, super-exponential  


  (1) <math> {\rm sex}_b(z)=\exp_b^z(1)</math>
  (1) <math>F_b(z)=\exp_b^z(1)</math>


is combination of <math>x</math> exponentials on base <math>b</math>. For example,
is combination of <math>z</math> exponentials on base <math>b</math>. For example,
: <math>~ {\rm sex}_b(0)=\exp_b^0(1)=1</math>
: <math>~ F_b(0)=\exp_b^0(1)=1</math>
: <math>~ {\rm sex}_b(1)=\exp_b(1)=b</math>
: <math>~ F_b(1)=\exp_b(1)=b</math>
: <math>~ {\rm sex}_b(2)=\exp_b^2(1)=\exp_b(\exp_b(1))=b^b</math>
: <math>~ F_b(2)=\exp_b^2(1)=\exp_b(\exp_b(1))=b^b</math>
: <math>~ {\rm sex}_b(3)=\exp_b^3(1)=\exp_b\Big(\exp_b\big(\exp_b(1)\big)\Big)=b^{b^b}</math>
: <math>~ F_b(3)=\exp_b^3(1)=\exp_b\Big(\exp_b\big(\exp_b(1)\big)\Big)=b^{b^b}</math>
and so on. However, such definition is good only for positive integer values of <math>x</math>. In general, the superexponential can be defined through the [[Abel equation]]
and so on. However, such definition is good only for positive integer values of <math>z</math>. In general, the superexponential can be defined through the [[Abel equation]]
  (2) <math>~\exp_b\Big({\rm sex_b}(z)\Big)={\rm sex}_b(z+1) </math>
  (2) <math>~\exp_b\Big(F_b(z)\Big)=F_b(z+1) </math>
with additional condition that  
with additional condition that  
  (3) <math>~{\rm sex_b}(0)=1</math>
  (3) <math>~F_b(0)=1</math>
Then, at least for positive values of <math>~b</math> and positive integer values of <math>~z</math>,
Then, at least for positive values of <math>~b</math> and positive integer values of <math>~z</math>,
such a definition can be used for the evaluation of tetration.  
such a definition can be used for the evaluation of tetration.  
Line 50: Line 51:
In this paper, the way to define tetration for non-integer argument is described. For real values of the argument, at <math>~b=2</math>, such a tetration is plotted on figure 0. In the following sections,
In this paper, the way to define tetration for non-integer argument is described. For real values of the argument, at <math>~b=2</math>, such a tetration is plotted on figure 0. In the following sections,
I describe, why is it so important, how to define the tetration for non-integer values of the argument, how can it be evaluated with high precision and why it is the only correct way to define analytic tetration.
I describe, why is it so important, how to define the tetration for non-integer values of the argument, how can it be evaluated with high precision and why it is the only correct way to define analytic tetration.
===Table of superfunctions===
There exist many basefuncitons such that the supercunctions can be expressed in the closed form.
For some function <math>F</math> such that both <math>F</math> and <math>F^{-1}</math>
can be expressed with simple and explicit formulas, the basefunciton
:<math>H(z)=F(1+H^{-1}(z))</math>.
In such a way, the table below can be constructed.


===Additional argument===
===Additional argument===
One can consider to add the additional argument, replacing  
One can consider to add the additional argument, replacing  
<math>~\exp_b(1)</math> to  
<math>~\exp_b(1)</math> to  
<math>~\exp_b(a)</math>. This may have sense, while <math>~z</math> is allowed to have only integer values. However, at the implementation of "good" tetration, the "argument" <math>~a</math> can be considered as inverse superexponential of some argument, <math>~a={\rm sex}_b(\alpha)</math>; then,
<math>~\exp_b(a)</math>. This may have sense, while <math>~z</math> is allowed to have only integer values. However, at the implementation of "good" tetration, the "argument" <math>~a</math> can be considered as inverse superexponential of some argument, <math>~a=F_b(\alpha)</math>; then,
<math>~\exp_b^z(a)={\rm sex}_b\Big({\rm sex}_b(\alpha) \Big)</math>; in the way, similar to that of convential logarithms: it is sufficient to investigate properties of natural logarithm ln; then, any other can be expressed as <math>\log_b(z)=\ln(z)/\ln(b)</math>.
<math>~\exp_b^z(a)=F_b\Big(F_b(\alpha) \Big)</math>; in the way, similar to that of convential logarithms: it is sufficient to investigate properties of natural logarithm ln; then, any other can be expressed as <math>\log_b(z)=\ln(z)/\ln(b)</math>.


  <math> \exp_b^n(\exp_b^m(z))=\exp_b^{m+n}(z) </math>
  <math> \exp_b^n(\exp_b^m(z))=\exp_b^{m+n}(z) </math>
Line 61: Line 69:
The exponentiation of tetration is equivalent to increment of its argument. While summaton operation forms the [[group]], exponentiation does too.
The exponentiation of tetration is equivalent to increment of its argument. While summaton operation forms the [[group]], exponentiation does too.


<math>\exp_b^m({\rm sex}_b(z))={\rm sex}_b(z+m)</math>
<math>\exp_b^m(F_b(z))=F_b(z+m)</math>
 
The generalization to non-integer values of <math>m</math> is possible.
While writing formal expressions (and assuming existence of the inverse function <math>F^{-1}</math>), the
specific preperties of exponential function are not used; and the
generalization is possible. Such generalization is described in article [[User:Dmitrii Kouznetsov/loginal]].


===Inverse function and group properties===
===Inverse function and group properties===
In this section, I write  
In this section, I write  
<math>~F</math> instead of <math>~{\rm sex}_b</math>
<math>~F</math> instead of <math>~F_b</math>
and
and
<math>~\exp(z)</math> instead of <math>~\exp_b(z)</math>;
<math>~\exp(z)</math> instead of <math>~\exp_b(z)</math>;
onitting indices. However, you may recover them at any moment.  
omitting indices. However, you may recover them at any moment.  


(I am not sure which notation is best. D.)
(I am not sure which notation is best. D.)
Line 97: Line 110:
===Ambiguity of the real-analytic extension===
===Ambiguity of the real-analytic extension===


==Asymptotic==
==[[Abel equation]]==
Assume, the tetration <math>F</math> is defined with the [[Abel equation]]
Assume, the tetration <math>F</math> is defined with the [[Abel equation]]


Line 105: Line 118:


  (11) <math> F(0)=1</math>
  (11) <math> F(0)=1</math>
==Asymptotic==
The analytic extension of tetration <math>~F(z)</math> is supposed to grow
fast along the real axis of the complex <math>z</math>-plane,
at least for some values of base <math>b</math>.
However, it has no need to grow infinitely in the direction of imaginary axis. For mathematics of computation, it would be better, if <math>F(x+{\rm i} y)</math> remains bounded at <math>y\rightarrow +\infty</math>. Consider this possibility.
Assume, there exist solution <math>F(z)</math> of equations
(10), (11), analytic in the  <math>\Re(z) \ge 0</math>, with, probably, countable number of cuts and singularities at  <math>\Re(z)<0</math>,
with exponential asymptotic behavior
(12) <math> F(z)=L+\varepsilon(z) + o \big(\varepsilon(z)^{2}\big) </math>
within some range,<!-- between cuts, $\Im(z)>0$, !-->
where
(13) <math> \varepsilon(z)=\exp(Qz+r) </math>,
<math>Q</math> and  <math>r</math> are fixed complex numbers,
and <math>L</math> is eigenvalue of logarithm, solution of equation
(14) <math> x=\log_{b}(x)</math>.
{{Image|ExampleEquationLog01.png|right|300px|FIg.1. Example of graphic solution of equation
<math>x=\log_b(x)</math> for
<math>b=\sqrt{2}</math> (two real solutions, <math>x=2</math> and <math>x=4</math>),
<math>b=\exp(1/\rm e)</math> (one real solution <math>x=\rm e</math>)
<math>b=2</math> (no real solutions).}}
Solutions of equation (14) are called [[fixed point]]s of logarithm.
Three examples of graphical solution of equation (14) are shown in figure 1 for
<math>b=\sqrt{2}</math>,
<math>b=\exp(1/\rm e)</math>,  and
<math>b=2</math>.
The black line shows function <math> y=x</math> in the <math>x,y</math> plane.
The colored curves show function <math> y=\log_b(x)</math> for cases
<math>b=\sqrt{2}</math>  (red),
<math>b=\exp(1/\rm e)</math> (green),  and
<math>b=2</math> (blue).
At
<math>b=\sqrt{2}</math>, there exist 2 solutions,
<math>x=2</math> and
<math>x=4</math>.
At
<math>b=\exp(1/\rm e)</math> there exist one solution
<math>x=\rm e</math>.
and <math>b=2</math>, there are no real solutions.<br>
In general,
*at <math>b<\exp(1/\rm e)</math> there are two real solutions
*at <math>b=\exp(1/\rm e)</math>, there is one soluition, and
*at <math>b>\exp(1/\rm e)</math> there esist two solutions, but they are complex.
In particular,
at
<math> b=\sqrt{2}</math>, the solutions are <br>
<math>x=L_{\sqrt{2},1}=2</math> and
<math>x=L_{\sqrt{2},2}=4 </math> <br>.
At
<math> b=2</math>, the solutions are <br>
<math>x=L_2 \approx 0.824678546142074222314065+1.56743212384964786105857 \!~\rm i </math> and<br>
<math>x=L_2^*\approx 0.824678546142074222314065-1.56743212384964786105857 \!~\rm i </math>.
At <math> b=\rm e</math>, the solutions are<br>
<math>x=L_{\rm e} \approx 0.318131505204764135312654+1.33723570143068940890116 \!~\rm i</math>
and<br>
<math>x=L_{\rm e}^* \approx 0.318131505204764135312654-1.33723570143068940890116 \!~\rm i</math>.
Few hundred straightforward iterations of equation (14) are sufficient to get the error smaller than the last decimal digit in the approximations above.
The solutions
<math>x=L_1          </math> and
<math>x=L_2          </math>  of equation (14) are plotted in figure 2 versus
<math>\beta=\ln(b)</math> with thin black lines. Let
<math>L_1<L_2      </math>, and only at
<math>\ln(b)=1/e  </math>, the equality
<math>L_1=L_2      </math> takes place.
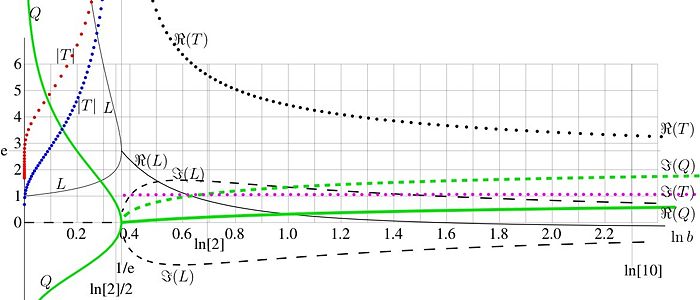
{{Image|TetrationAsymptoticParameters01.jpg|right|700px|FIg.2. parameters of asymptotic of tetration versus logarithm of the base}}
The thin black solid curve at
<math> \beta \ge 1/\rm e</math> represents the real part of the solutions
<math>L                          </math> and
<math>L^*                      </math> of (14); the thin black dashed curve represents the two options for the imaginary part; the two solutions are complex conjugaitons of each other. Let
<math>\Re(L)>0</math>.
===Increment and periodicity===
Parameter
<math> Q </math> in equaiton (13) has sense of asymptotic increment. Consider possible values of  <math>Q</math>.
Substitution of expression (13) into the eq. (12) gives<br>
<math> L+\varepsilon(z) {\rm e}^Q+o(\varepsilon(z))=\exp\!\Big(\ln(b)(L+\varepsilon(z)+o(\varepsilon(z))\Big) </math>
Using equation (14) gives<br>
<math> L+\varepsilon(z){\rm e}^Q+o(\varepsilon(z)) = L~\Big(1+ \ln(b)\varepsilon(z) +o(\varepsilon(z))\Big) </math>
Then
(16) <math> Q=\ln(\ln(b))+\ln(L) </math>.
At 
<math>\ln(b)<1/\rm e  </math>, the two real increments correspond the the two real eigenvalues of logarithm. Let
<math> Q_1=\ln(\ln(b))+\ln(L_1) </math> and
<math> Q_2=\ln(\ln(b))+\ln(L_2) </math>.
Both
<math> Q_1        </math> and <math> Q_2        </math> are plotted in Figure 2 with thick solid lines;
<math> Q_1\le 0 </math> and  <math> Q_2\ge 0 </math>, and only at
<math>\ln(b)=1/\rm e      </math> the equality
<math> Q_1= Q_2= 0 </math> holds.
In such a way, at <math>\ln(b)<1/\rm e</math>, there exist positive increment, which corresponds to <math>L_2</math> and means, that the perturbation <math>\epsilon(z)</math> of the stationary solution grows up in the diredtion of real axis; there exist negative increment (decrement, if you like), which corresponds to <math>L_1</math> and indicates, that the perturbation grows up in the opposite direction.
At <math>0<\ln(b)<2/\rm e</math>, there may exist tetration <math> F(z)</math> with two different asumptotics.
At large positive values of <math>\Re(z)</math> it decays to the  <math>L_1</math> and
At large negative values of <math>\Re(z)</math> it decays to the  <math>L_2</math>.
At
<math>\ln(b)\ge 1/\rm e    </math>, there exist two possible increments,
<math>Q</math> and <math>Q^*</math>. The real part of <math>Q</math> is plotted with thick solid line, and the imaginary part is shown with thick dashed line. The real part is positive; id est, the perturbation
<math>\varepsilon(z)</math> grows up in the direction of real axis.
For computational mathematics, it would be good ot construct the solution, that decays both in the direction of the imaginary asis and in the opposite direction. Such a solution should have asymptotics (12),(13) at large positive values of the imaginary part of the argument and its conjugation at large negative imaginary part of the argument.
The exponential asymptotics implies the asumptotic perioditity. The period
<math>T=\frac{2 \pi{\rm i}}{Q} </math>
is shown in FIgure 2 with dotted lines; for the case when period is negative, the minus period is plotted. It is amaising, that at <math>\ln(b)>1/\rm e</math>, the imajinary part is slightly larger than unity and remains almost constant.
In particular, at <math>b=\sqrt{2}</math>, the periods are
<math> T_{\rm b,1} = \frac{2\pi \rm i}{Q_{b,1}}\approx -17.143148179354847104 \!~\rm i
</math><br>
<math> T_{\rm b,2} = \frac{2\pi \rm i}{Q_{b,2}}\approx ~ 19.236149042042854710 \!~\rm i
</math><br>
At
<math> b=2</math>, the asymptotic period<br>
<math> T= 5.58414243554338946020010 + 1.05421836033693734654000\!~ \rm i</math>;
<br>
and  at
<math>b=\rm e</math>, the asymptotic period<br>
<math> T=  4.44695072006700782711227 + 1.05793999115693918376341 \!~ \rm i</math>.


While one deal with real axis, one imagine some real-analytic extension shown in Figure 0 and consider also  
===Uniqueness===
While one deal with solutions of system (10), (11) at the real axis, one imagine some real-analytic extension shown in Figure 0 and consider also  


  (12) <math> G(z)=F(z+k(z))</math>
  (100) <math> G(z)=F(z+k(z))</math>


where
where


  (13) <math> k(z)=\sum_{n=-\infty}^{\infty} \alpha_n \exp(2\pi {\rm i} n z)</math>
  (101) <math> k(z)=\sum_{n=-\infty}^{\infty} \alpha_n \exp(2\pi {\rm i} n z)</math>


Such a funciton is also coluiton of the Abel equation; at  
Such a funciton is also soluiton of the Abel equation; at  


  (14) <math> \alpha_{-n}=(\alpha_n)^* </math>
  (102) <math> \alpha_{-n}=(\alpha_n)^* </math>


and
and


  (15) <math> \alpha_0=-\sum_{n\ne 0} \alpha_n</math>
  (103) <math> \alpha_0=-\sum_{n\ne 0} \alpha_n</math>


function <math>~G</math> is real and passes through the same points as </math>~F</math> at integer values of the argument.
function <math>~G</math> is real and passes through the same points as </math>~F</math> at integer values of the argument.
Line 130: Line 280:
However, the difference between functions  
However, the difference between functions  
<math>~F</math> and
<math>~F</math> and
<math>~G</math> nefomes seen, if one of them, for example, <math>~F</math>, is analytic and regular in the upper and lower half-planes;
<math>~G</math> becomes seen, if one of them, for example, <math>~F</math>, is analytic and regular in some wide range, function <math>~G(z)</math> will be analytic only within the strip <math>~|z|<y_0</math>; order of magnitude of   
function <math>~G(z)</math> will be analytic only within the strip <math>~|z|<y_0</math>; order of magnitude of   
<math>~y_0</math> can be estimated with
<math>~y_0</math> can be estimated with


<math>y_0 \approx {\rm MIN}_n ~ \frac{ \ln(2/|\alpha_n|)}{2\pi n}</math>
<math>y_0 \approx {\rm MIN}_n ~ \frac{ \ln(2/|\alpha_n|)}{2\pi n}</math>


At larger values of the imaginary part of the arguemnt, the periodic function <math>k</math> takes huge values, including various negative integers. Namely at these values, function <math>~F</math> has singulatities.
At larger values of the imaginary part of the argument, the periodic function <math>k</math> takes huge values, including various negative integers. Namely at these values, function <math>~F</math> has singulatities.
 
For abstract excersize in comlex functional analysis,[[all animals are equal]]. However, for the applicaitons in the computaional mathematics, [[some of them are more equal than other]].
As such a ''more equal'' animal we should choose the function with simplest behavior, with minimum of singulatities, and easiest for the evaluation.
 
===Eigenvalues of logarithm===
[[Image:ExampleEquationLog01.png|300px|right|thumb|FIg.1. Example of graphic solution of equation <math>L=\log_b(L)</math> for
<math>b=\sqrt{2}</math> (two real solutions, <math>L=2</math> and <math>L=4</math>),
<math>b=\exp(1/\rm e)</math> (one real solution <math>L=\rm e</math>)
<math>b=2</math> (no real solutions).]]
 
Function <math>F</math> is supposed to grow up (faster than any exponential) in the direction of the real axis.
However, it has no need to grow in other directions; neither in the opposite direction, nor in the direction of the imaginary axis.
 
For computational mathematics, it would be better, if it does not grow at all, approaching some limiting value.
Also, for computation, it would be better, if all the signulatities of function <math>~F</math> are at the real axis; at values <math>-2</math> and less (more negative).
 
There is not so much opportunity in choise of this limiting value. From the equaiton of Abel, it follows, that the asymptotics should be eigenvalue of logarithm, i.e., solution
<math>~L </math> of equation


(20) <math> L=\log_b (L) </math>
Various functions may satisfy equations (2),(3).
 
For an abstract excersise in comlex functional analysis, [[all animals are created equal]]. However, for the applicaitons in the computational mathematics, [[some of them are more equal than other]]
Figure 1 shows the graphic of the left-hand (blask) and the of this equation (black line) and 3 examples of the right-hand side.
<ref name="orwell">'''All animals are created equal''' and that with addition '''BUT SOME ANIMALS ARE MORE EQUAL THAN OTHERS''' are slogans of animals in the novel [[Animal farm]] by George Orwell. (Animals gained the superior power at the farm, bushing out the farmer). First slogan was used to gain the power. The second part appeared when pigs begun to justify their privilegies.</ref>.
 
As such a ''more equal animal'' we should choose the solution with simplest behavior, with minimum of singulatities, and easiest for the evaluation.
Two real solutions  exist at <math>~b< \exp(1/\rm e)</math>. These solutions are plotted versus  <math>~\ln(b) </math> in Figure 2 with thin black solid line.
 
One real solution <math>~L=\rm e</math> exists at  <math>~b= \exp(1/\rm e)</math>.
 
Two complex solutions exist at <math>~b> \exp(1/\rm e)</math>. These solutions are mutially conjugated. The real part is plotted with solid line, and two options for the imaginary part are plotted with dashed line.


<!--
<!--
These solutions are plotted versus <math>~\ln(b) </math> in Figure 2.
I search for the simplest solution, that  asymptotically, approaches the eigenvalue of logarithm. For <math>b</math> slightly larger than unity, there exist real sloutions, as it is shown in figure 1.
For <math>b>\exp(1/\rm e)</math>, there are 2 complex solutions shown in figure 2.
!-->
[[Image:TetrationAsymptoticParameters00.jpg|700px|right|thumb|FIg.2. parameters of asymptotic of tetration versus logarithm of the base]]
==Exponential asymptotic==
==Exponential asymptotic==
Consider tetration with following asymptotic behavior:
Consider tetration with following asymptotic behavior:
Line 185: Line 305:


Quasi-period <math>T=2\pi/Q</math> is plotted with dotted lines.  
Quasi-period <math>T=2\pi/Q</math> is plotted with dotted lines.  
!-->


At small base, both values of quasiperiod are pure imaginary. This periodic or quasi-periodic behavior is similar to that of the conventional exponential,  
At small base, both values of quasiperiod are pure imaginary. This periodic or quasi-periodic behavior is similar to that of the conventional exponential,  
Line 192: Line 313:
At <math>~b> \exp(1/{\rm e})</math> values of period <math>T</math> are complex; and  
At <math>~b> \exp(1/{\rm e})</math> values of period <math>T</math> are complex; and  
<math>\Re(T)>0</math>. This corresponds to the exponential growth of the asymptotic solution in the direction of the real axis; at large positive values of <math>\Re(Qz)</math> the asymptotic does not approximate the function which grows faster than any exponential.
<math>\Re(T)>0</math>. This corresponds to the exponential growth of the asymptotic solution in the direction of the real axis; at large positive values of <math>\Re(Qz)</math> the asymptotic does not approximate the function which grows faster than any exponential.
==Examples of tetration at different bases==
===Small base. Base <math>b=\sqrt{2}</math>===
===Small base. Base <math>b=\sqrt{2}</math>===
[[Image:AnalyticTetrationBaseSqrt2v00.jpg|800px|right|thumb|Fig.3. Tetration <math>f=F(z)</math> at the complex <math>z</math>-plane.
{{Image|AnalyticTetrationBaseSqrt2v00.jpg|right|220px|Fig.3a. Tetration <math>f=F(z)</math> at the complex <math>z</math>-plane.
Levels of integer <math>\Re(f)</math> and levels of integer <math>\Im(f)</math> are shown with thick lines.
Levels of integer <math>\Re(f)</math> and levels of integer <math>\Im(f)</math> are shown with thick lines.
Grid covers with unit step range  
<!--Grid covers with unit step range  
(<math>-10\le \Re(z) \le 10 </math>,
(<math>-10\le \Re(z) \le 10 </math>,
<math>-10\le \Im(z) \le 10 </math>).]]
<math>-10\le \Im(z) \le 10 </math>).!-->}}


For evaluation of Tetration we need to assume that it exists, and has asymptotic (30).
For evaluation of Tetration we need to assume that it exists, and has asymptotic (30).
Line 205: Line 328:
the function has periodic behavior in the direction of imaginary axis, as it is shown in Fig. 3. In this figure, the example with <math>b=\sqrt{2}</math> is used. Function is periodic; period <math>T\approx 17.1431~\rm i</math>.
the function has periodic behavior in the direction of imaginary axis, as it is shown in Fig. 3. In this figure, the example with <math>b=\sqrt{2}</math> is used. Function is periodic; period <math>T\approx 17.1431~\rm i</math>.


As all analytic tetrations, function has limiting values;
The limiting values:


<math> \lim_{x \rightarrow \infty} F_\sqrt{2}(x+{\rm i}y)=2</math>
<math> \lim_{x \rightarrow \infty} F_\sqrt{2}(x+{\rm i}y)=2</math>


<math> \lim_{x \rightarrow -\infty} F_\sqrt{2}(x+{\rm i}y)=4</math>
<math> \lim_{x \rightarrow -\infty} F_\sqrt{2}(x+{\rm i}y)=4</math>


At non-zero values of the imaginary part of the argument, the function decays  
At non-zero values of the imaginary part of the argument, the function decays  
Line 222: Line 345:
As all other tetrations, it has singularity at <math>z=-2</math>,  
As all other tetrations, it has singularity at <math>z=-2</math>,  
<math>~F(-1)=0</math> and <math>~F(0)=1</math>.
<math>~F(-1)=0</math> and <math>~F(0)=1</math>.
===Base <math>b=\sqrt{2}</math>, alternative tetration <math>G</math>===
{{Image|AnalyticTetrationBaseSqrt2u00.png|left|240px|Fig.3b. Tetration <math>f=G(z)</math> at the complex plane <math>z=x+{\rm i}y</math>.
Levels of integer <math>\Re(f)</math> and levels of integer <math>\Im(f)</math> are shown with thick lines.
<!--Grid covers with unit step range
(<math>-10\le \Re(z) \le 10 </math>,
<math>-10\le \Im(z) \le 10 </math>).!-->}}
Along the real axis, such a solution grows up faster than any exponential; it is always larger than 4 and therefore cannot be equal to unity; so, it is not possible to satisfy condition <math>G(0)=0</math> just shifting its argument for some constant. The behavior of tetration <math>G_{\sqrt{2}}</math> along the real axis makes it similar to that of tetrations with <math>b > \exp(1/\rm e)</math>; although, the larger
<math>b</math>, the larger is the growth.
===More alternative tetrations===
Alternative tetration <math> G </math> as solution of equation (1) can be calculated if we withdraw condition (2), and substitute it to
<math>
G(z)=L_{b,2}+\exp(Q_2 z)+o( \exp(Q_2 z))
</math>
Such a solution is shown in Figure 3b.


Other, more singular tetrations can be obtained by light periodic deformation of the argument.
Other, more singular tetrations can be obtained by light periodic deformation of the argument.


=== Base 2. Ackermann function===
=== Base 2. Ackermann function===
[[Image:Analytic4thAckermannFunction00.jpg|600px|right|thumb| FIg.4.
{{Image|Analytic4thAckermannFunction00.jpg|right|600px| FIg.4.
Ackermann function <math>f=A(4,z)=F_2(z+3)-3</math> at the complex <math>z</math> plane.
Ackermann function <math>f=A(4,z)=F_2(z+3)-3</math> at the complex <math>z</math> plane.
Grid covers the range (
Grid covers the range (
Line 232: Line 372:
-10 \le \Re(z) \le 10 ~,~
-10 \le \Re(z) \le 10 ~,~
  -4 \le \Im(z) \le  4 </math> with unit step.
  -4 \le \Im(z) \le  4 </math> with unit step.
Levels of integer values of the real part and the same for imaginary part are shown with thick lines.]]
Levels of integer values of the real part and the same for imaginary part are shown with thick lines.}}


Tertration at base 2 can be expressed through the 4th Ackermann function
Tertration at base 2 can be expressed through the 4th [[Ackermann function]]
<math>F_2(z)=A(4,z-3)+3</math>
<math>F_2(z)=A(4,z-3)+3</math>
This Ackermann function is plotted in fig.4. As base  
This Ackermann function is plotted in fig.4. As base  
<math>b > \exp(1/\rm e)</math>, the function is not periodic. However, there is asymptotic periods
<math>b > \exp(1/\rm e)</math>, the function is not periodic. However, there are asymptotic periods
<math>L</math> and <math>L^*</math>
<math>L</math> and <math>L^*</math>
in the upper and lower half-planes. In vicinity of positive part of the real axis, the function shows rapid growth, and it is not possible to draw the levels there. All the eingularities are at the negative integer values smaller than -1.
in the upper and lower half-planes. In vicinity of positive part of the real axis, the function shows rapid growth, and it is not possible to draw the levels there. All the singularities of the Ackermann function are at the negative integer values smaller than <math>-4</math>.


=== Base e. Natural tetration ===
=== Base e. Natural tetration ===
Line 255: Line 395:
Base <math>b-\rm e</math> is the most natural choise. In this case, the  
Base <math>b-\rm e</math> is the most natural choise. In this case, the  
increment <math>Q</math> is equal to the asymtotic value <math>L</math>.
increment <math>Q</math> is equal to the asymtotic value <math>L</math>.


At the translation for inity along the real axis,
At the translation for inity along the real axis,


===Superlogarithm===
===Base e. Superlogarithm===
[[Image:Slogez01.jpg|300px|right|thumb|Fig.7. Inverse of the analytic tetration  
{{Image|Slogez01.jpg|left|300px|Fig.7. Inverse of the analytic tetration  
<math>f=F_{\rm e}^{-1}(z)</math> at the complex <math>z</math>-plane.]]
<math>f=F_{\rm e}^{-1}(z)</math> at the complex <math>z</math>-plane.}}


Inverse of the natural tetration can be considered as superlogarithm.
Inverse of the natural tetration can be considered as superlogarithm.
Line 283: Line 421:
<references/>
<references/>
See also the discussion at http://math.eretrandre.org/tetrationforum/forumdisplay.php?fid=3
See also the discussion at http://math.eretrandre.org/tetrationforum/forumdisplay.php?fid=3
[[Category:Mathematics]]
 
[[Category:Mathematical analysis]]
===Table of superfunctions===
[[Category:Funcitons]]
There exist many basefuncitons such that the supercunctions can be expressed in the closed form.
[[Category:tetration]]
For some function <math>F</math> such that both <math>F</math> and <math>F^{-1}</math>
can be expressed with simple and explicit formulas, the basefunciton
:<math>H(z)=F(1+F^{-1}(z))</math>.
In such a way, the table below can be constructed.
{|class="wikitable"
! <math>H(z)</math>
! <math>F(z)</math>
! <math>F^{-1}(z)</math>
! comment
|-
|<math>z+\!\!+</math>||<math>b+z</math>||<math>z-b</math> ||
|-
|<math>z+b</math>||<math>bz+c</math> || <math>(z-c)/b</math> ||
|-
|<math>cz+c</math>||<math>(b^z+\frac{c}{1-b})d</math>||<math>\log_b\frac{z-\frac{cd}{1-b}}{d}</math>||
|-
|<math>b^z</math> || <math>\mathrm{tet}_b(z)</math> || <math>\mathrm{arctet}_b(z)</math>||[[tetration]]
|-
|<math>z^b</math>|| <math>\exp(b^z)</math> || <math>\log_b(\ln(z))</math>||
|-
|<math>\big(a^b+z^b\big)^{1/b}</math>||<math>az^{1/b}</math>||<math>(z/a)^b</math>||
|-
|<math>\ln(a+\mathrm{e}^z)</math>||<math>\ln(az)</math>||<math>\exp(z)/a</math>||
|-
|<math>az/(z+a)</math>||<math>a/z</math>||<math>a/z</math>||amaising, <math>F=F^{-1}</math>
|-
|<math>2z^2-1</math>||<math>\cos(\pi 2^z)</math>||<math>\log_2(\arccos(z)/\pi)</math>||
|-
|<math>H(z)</math>||<math>F(z)</math>||<math>F^{-1}(z)</math>||
|-
|<math>P(H(Q(z)))</math>||<math>P(F(z))</math>||<math>F^{-1}(Q(z))</math>|| P(Q(z))=z
|-|}

Latest revision as of 10:20, 14 June 2024


The account of this former contributor was not re-activated after the server upgrade of March 2022.


Fig.0. Graphic of analytic tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=F_2(x)=\exp_2^x(1)} versus .

In this page, I collect pieces and figures, which can be used to build-up tetration.

Abstract

Analytic tetration is defined as mathematical function that coincides witht the tetration at integer values of the argument and is analytic outside the negative part of the real axis. Existence of such a function is postulated; and arguments in favor of uniqueness of such a function are considered. The algorithm of evaluation is suggested. Examples of evaluation, pictures and tables are supplied. The application and the generalization is discussed.

Preface

The colleagues indicated so many misprints in my papers about tetration, posted at my homepage [1], that I want to give them opportunity to correct them in real time.

Especially I invite

  • Arthur Knoebel
  • Henryk Trappmann
  • Andrew Robbins

to edit this file.

I consider the topic very important and urgent. The analytic tetration should be investigated and discussed right now; overvice, the non-analytic extension may become an ugly standard in mathematics of computation; the implementation of hige numbers with non-analytic tetration would make difficult realization of arithmetic operations and cause a lot of incompatibilities.

This is my apology for posting this research now, while the rigorous proof of existence and uniqueness of the analytic tetration is not yet found. My believe is based on the numerical check of the hypothesis of the existence and uniqueness, on smallness of the residual at the substitution of the function to the tetration equation and beauty of the resulting pictures. I cannot imagine that the agreement with 14 decimal digits occurs just by occasion without deep mathematical meaning.

In such a way I apologize for postulating of statements which should be prooven by the rigorous mathematical deduction.

Introduction

Quick start

Roughly, super-exponential 

(1) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_b(z)=\exp_b^z(1)}

is combination of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} exponentials on base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} . For example,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~ F_b(0)=\exp_b^0(1)=1}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~ F_b(1)=\exp_b(1)=b}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~ F_b(2)=\exp_b^2(1)=\exp_b(\exp_b(1))=b^b}

and so on. However, such definition is good only for positive integer values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} . In general, the superexponential can be defined through the Abel equation 

(2)

with additional condition that 

(3) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F_b(0)=1}

Then, at least for positive values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~b} and positive integer values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~z} , such a definition can be used for the evaluation of tetration.

In this paper, the way to define tetration for non-integer argument is described. For real values of the argument, at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~b=2} , such a tetration is plotted on figure 0. In the following sections, I describe, why is it so important, how to define the tetration for non-integer values of the argument, how can it be evaluated with high precision and why it is the only correct way to define analytic tetration.

Table of superfunctions

There exist many basefuncitons such that the supercunctions can be expressed in the closed form. For some function such that both Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F^{-1}} can be expressed with simple and explicit formulas, the basefunciton

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H(z)=F(1+H^{-1}(z))} .

In such a way, the table below can be constructed.

Additional argument

One can consider to add the additional argument, replacing to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~\exp_b(a)} . This may have sense, while is allowed to have only integer values. However, at the implementation of "good" tetration, the "argument" Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~a} can be considered as inverse superexponential of some argument, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~a=F_b(\alpha)} ; then, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~\exp_b^z(a)=F_b\Big(F_b(\alpha) \Big)} ; in the way, similar to that of convential logarithms: it is sufficient to investigate properties of natural logarithm ln; then, any other can be expressed as Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \log_b(z)=\ln(z)/\ln(b)} . 

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  \exp_b^n(\exp_b^m(z))=\exp_b^{m+n}(z) }

The exponentiation of tetration is equivalent to increment of its argument. While summaton operation forms the group, exponentiation does too.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \exp_b^m(F_b(z))=F_b(z+m)}

The generalization to non-integer values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle m} is possible. While writing formal expressions (and assuming existence of the inverse function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F^{-1}} ), the specific preperties of exponential function are not used; and the generalization is possible. Such generalization is described in article User:Dmitrii Kouznetsov/loginal.

Inverse function and group properties

In this section, I write Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F} instead of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F_b} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~\exp(z)} instead of ; omitting indices. However, you may recover them at any moment.

(I am not sure which notation is best. D.)

The speculation of the previous subseciton can be written shorter.

Assume there exist function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F^{-1}} such that Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~ F\Big(F^{-1}(z)\Big)=z} .

Let Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~s=\exp^u(t)=F\Big(u+F^{-1}(t)\big)} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~q=\exp^v(s)=F\Big(v+F^{-1}(s)\big)} .

Then Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~q =\exp^v\Big( \exp^u(t) \Big) =F\Big(v+F^{-1}\Big(F(u+F^{-1}(t))\Big)\Big) =F\Big(v+u+F^{-1}(t)\Big) } .

You can put subscript Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~_b} to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F} and in the defuction above, and it will be seen, that we have no need to deal with funciton of 2 variables, considering Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \exp_b^z(x)} ; it can be expressed in terms of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_b(z)} . However, we need to specify, what set shold be Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~t} and from in the deduction above: must they be positive integer, or they can be real, of they can be also complex numbers.

History of tetration and huge numbers

Perhaps, every researcher used to see diagnostivs "floating overflow" at the evlauation of an expression with huge numbers....

Ackermann functions

Ambiguity of the real-analytic extension

Abel equation

Assume, the tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F} is defined with the Abel equation 

(10) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  \exp_b(F(z))=F(z+1)}

and assume the condition 

(11) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  F(0)=1}

Asymptotic

The analytic extension of tetration is supposed to grow fast along the real axis of the complex Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} -plane, at least for some values of base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} . However, it has no need to grow infinitely in the direction of imaginary axis. For mathematics of computation, it would be better, if Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F(x+{\rm i} y)} remains bounded at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y\rightarrow +\infty} . Consider this possibility.

Assume, there exist solution Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F(z)} of equations (10), (11), analytic in the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(z) \ge 0} , with, probably, countable number of cuts and singularities at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(z)<0} , with exponential asymptotic behavior 

(12) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  F(z)=L+\varepsilon(z) + o \big(\varepsilon(z)^{2}\big) }

within some range, where 

(13) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  \varepsilon(z)=\exp(Qz+r) }
,

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle r} are fixed complex numbers, and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L} is eigenvalue of logarithm, solution of equation 

(14) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  x=\log_{b}(x)}
.
© Image: Dmitrii Kouznetsov
FIg.1. Example of graphic solution of equation Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=\log_b(x)} for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} (two real solutions, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=2} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=4} ), Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\exp(1/\rm e)} (one real solution Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=\rm e} ) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} (no real solutions).

Solutions of equation (14) are called fixed points of logarithm. Three examples of graphical solution of equation (14) are shown in figure 1 for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\exp(1/\rm e)} , and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} .

The black line shows function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=x} in the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x,y} plane. The colored curves show function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y=\log_b(x)} for cases Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} (red), Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\exp(1/\rm e)} (green), and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} (blue).

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} , there exist 2 solutions, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=2} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=4} .

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\exp(1/\rm e)} there exist one solution Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=\rm e} .

and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} , there are no real solutions.

In general,

  • at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b<\exp(1/\rm e)} there are two real solutions
  • at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\exp(1/\rm e)} , there is one soluition, and
  • at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b>\exp(1/\rm e)} there esist two solutions, but they are complex.

In particular, at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} , the solutions are
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_{\sqrt{2},1}=2} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_{\sqrt{2},2}=4 }
.

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} , the solutions are
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_2 \approx 0.824678546142074222314065+1.56743212384964786105857 \!~\rm i } and
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_2^*\approx 0.824678546142074222314065-1.56743212384964786105857 \!~\rm i } .

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\rm e} , the solutions are
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_{\rm e} \approx 0.318131505204764135312654+1.33723570143068940890116 \!~\rm i} and
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_{\rm e}^* \approx 0.318131505204764135312654-1.33723570143068940890116 \!~\rm i} .

Few hundred straightforward iterations of equation (14) are sufficient to get the error smaller than the last decimal digit in the approximations above.

The solutions Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_1 } and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle x=L_2 } of equation (14) are plotted in figure 2 versus Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \beta=\ln(b)} with thin black lines. Let Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_1<L_2 } , and only at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(b)=1/e } , the equality Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_1=L_2 } takes place.

FIg.2. parameters of asymptotic of tetration versus logarithm of the base

The thin black solid curve at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \beta \ge 1/\rm e} represents the real part of the solutions Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L } and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L^* } of (14); the thin black dashed curve represents the two options for the imaginary part; the two solutions are complex conjugaitons of each other. Let Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(L)>0} .

Increment and periodicity

Parameter Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q } in equaiton (13) has sense of asymptotic increment. Consider possible values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} .

Substitution of expression (13) into the eq. (12) gives
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L+\varepsilon(z) {\rm e}^Q+o(\varepsilon(z))=\exp\!\Big(\ln(b)(L+\varepsilon(z)+o(\varepsilon(z))\Big) } Using equation (14) gives

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  L+\varepsilon(z){\rm e}^Q+o(\varepsilon(z)) = L~\Big(1+ \ln(b)\varepsilon(z) +o(\varepsilon(z))\Big) }

Then 

(16) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  Q=\ln(\ln(b))+\ln(L) }
.

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(b)<1/\rm e } , the two real increments correspond the the two real eigenvalues of logarithm. Let Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_1=\ln(\ln(b))+\ln(L_1) } and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_2=\ln(\ln(b))+\ln(L_2) } . Both Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_1 } and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_2 } are plotted in Figure 2 with thick solid lines; Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_1\le 0 } and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_2\ge 0 } , and only at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(b)=1/\rm e } the equality Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q_1= Q_2= 0 } holds.

In such a way, at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(b)<1/\rm e} , there exist positive increment, which corresponds to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_2} and means, that the perturbation Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \epsilon(z)} of the stationary solution grows up in the diredtion of real axis; there exist negative increment (decrement, if you like), which corresponds to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_1} and indicates, that the perturbation grows up in the opposite direction. At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 0<\ln(b)<2/\rm e} , there may exist tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F(z)} with two different asumptotics. At large positive values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(z)} it decays to the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_1} and At large negative values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(z)} it decays to the Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L_2} .

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(b)\ge 1/\rm e } , there exist two possible increments, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q^*} . The real part of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} is plotted with thick solid line, and the imaginary part is shown with thick dashed line. The real part is positive; id est, the perturbation Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \varepsilon(z)} grows up in the direction of real axis.

For computational mathematics, it would be good ot construct the solution, that decays both in the direction of the imaginary asis and in the opposite direction. Such a solution should have asymptotics (12),(13) at large positive values of the imaginary part of the argument and its conjugation at large negative imaginary part of the argument.

The exponential asymptotics implies the asumptotic perioditity. The period 

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T=\frac{2 \pi{\rm i}}{Q} }

is shown in FIgure 2 with dotted lines; for the case when period is negative, the minus period is plotted. It is amaising, that at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(b)>1/\rm e} , the imajinary part is slightly larger than unity and remains almost constant.

In particular, at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} , the periods are

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T_{\rm b,1} = \frac{2\pi \rm i}{Q_{b,1}}\approx -17.143148179354847104 \!~\rm i }
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T_{\rm b,2} = \frac{2\pi \rm i}{Q_{b,2}}\approx ~ 19.236149042042854710 \!~\rm i }


At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} , the asymptotic period
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T= 5.58414243554338946020010 + 1.05421836033693734654000\!~ \rm i} ;
and at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\rm e} , the asymptotic period
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T= 4.44695072006700782711227 + 1.05793999115693918376341 \!~ \rm i} .

Uniqueness

While one deal with solutions of system (10), (11) at the real axis, one imagine some real-analytic extension shown in Figure 0 and consider also 

(100) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  G(z)=F(z+k(z))}

where 

(101) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  k(z)=\sum_{n=-\infty}^{\infty} \alpha_n \exp(2\pi {\rm i} n z)}

Such a funciton is also soluiton of the Abel equation; at 

(102) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  \alpha_{-n}=(\alpha_n)^* }

and 

(103) Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle  \alpha_0=-\sum_{n\ne 0} \alpha_n}

function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~G} is real and passes through the same points as </math>~F</math> at integer values of the argument.

and at small values of coefficients Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \alpha} , function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~G} looks smooth, and it is difficult to guess, which of them is "true". For this reason, for the standard mathematical representation, the non-analytic stepwice funciton uxp was suggested [2].

However, the difference between functions Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~G} becomes seen, if one of them, for example, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F} , is analytic and regular in some wide range, function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~G(z)} will be analytic only within the strip Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~|z|<y_0} ; order of magnitude of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~y_0} can be estimated with

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle y_0 \approx {\rm MIN}_n ~ \frac{ \ln(2/|\alpha_n|)}{2\pi n}}

At larger values of the imaginary part of the argument, the periodic function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle k} takes huge values, including various negative integers. Namely at these values, function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F} has singulatities.

Various functions may satisfy equations (2),(3). For an abstract excersise in comlex functional analysis, all animals are created equal. However, for the applicaitons in the computational mathematics, some of them are more equal than other [3]. As such a more equal animal we should choose the solution with simplest behavior, with minimum of singulatities, and easiest for the evaluation.


At small base, both values of quasiperiod are pure imaginary. This periodic or quasi-periodic behavior is similar to that of the conventional exponential, but function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F} does not grow to infinity in the direction of the real axis, aproaching eigenvalue Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L} of logarithm. One example of such a behavior Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~b=\sqrt{2} } is shown in Figure 3 for Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~b=\sqrt{2}} . The following section describes, how it was ploted.

At Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~b> \exp(1/{\rm e})} values of period Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T} are complex; and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(T)>0} . This corresponds to the exponential growth of the asymptotic solution in the direction of the real axis; at large positive values of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(Qz)} the asymptotic does not approximate the function which grows faster than any exponential.

Examples of tetration at different bases

Small base. Base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}}

Fig.3a. Tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=F(z)} at the complex Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} -plane. Levels of integer Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(f)} and levels of integer Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Im(f)} are shown with thick lines.

For evaluation of Tetration we need to assume that it exists, and has asymptotic (30).

Consider the case of Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 1 < b < \exp(1/\rm e)} . at fixed values of real part of the argument, the function has periodic behavior in the direction of imaginary axis, as it is shown in Fig. 3. In this figure, the example with Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} is used. Function is periodic; period Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle T\approx 17.1431~\rm i} .

The limiting values:

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \lim_{x \rightarrow \infty} F_\sqrt{2}(x+{\rm i}y)=2}

At non-zero values of the imaginary part of the argument, the function decays to these asymptotiv values.

At the real axis, the has cut at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z\le -2} .

Due to the periodicity, the function has cuts also at

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Z+T n \le -2} for integer Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~n} .

As all other tetrations, it has singularity at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z=-2} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F(-1)=0} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle ~F(0)=1} .

Base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} , alternative tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G}

Fig.3b. Tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=G(z)} at the complex plane Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z=x+{\rm i}y} . Levels of integer Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(f)} and levels of integer Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Im(f)} are shown with thick lines.

Along the real axis, such a solution grows up faster than any exponential; it is always larger than 4 and therefore cannot be equal to unity; so, it is not possible to satisfy condition Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G(0)=0} just shifting its argument for some constant. The behavior of tetration along the real axis makes it similar to that of tetrations with ; although, the larger Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b} , the larger is the growth.

More alternative tetrations

Alternative tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G } as solution of equation (1) can be calculated if we withdraw condition (2), and substitute it to Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle G(z)=L_{b,2}+\exp(Q_2 z)+o( \exp(Q_2 z)) } Such a solution is shown in Figure 3b.

Other, more singular tetrations can be obtained by light periodic deformation of the argument.

Base 2. Ackermann function

FIg.4. Ackermann function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=A(4,z)=F_2(z+3)-3} at the complex Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} plane. Grid covers the range ( Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -10 \le \Re(z) \le 10 ~,~ -4 \le \Im(z) \le 4 } with unit step. Levels of integer values of the real part and the same for imaginary part are shown with thick lines.

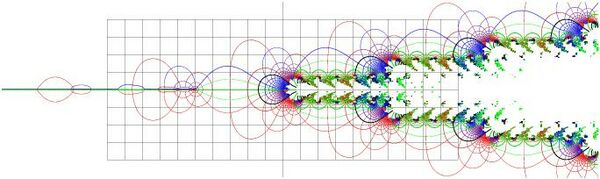
Tertration at base 2 can be expressed through the 4th Ackermann function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F_2(z)=A(4,z-3)+3} This Ackermann function is plotted in fig.4. As base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b > \exp(1/\rm e)} , the function is not periodic. However, there are asymptotic periods Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L^*} in the upper and lower half-planes. In vicinity of positive part of the real axis, the function shows rapid growth, and it is not possible to draw the levels there. All the singularities of the Ackermann function are at the negative integer values smaller than Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -4} .

Base e. Natural tetration

Fig.5.Analytic tetration at the base e , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=F_{\rm e}(z) } in the complex Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} plane. The grid covers the range (Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -9 \le \Re(z) \le 9} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -4 \le \Im(z) \le 4} ). Levels and levels Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle {\rm Arg}(f)=0, \pm 1, \pm 2, \pm3 } are shown with thick lines.

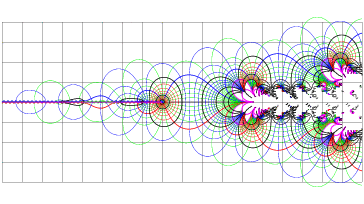
Base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b-\rm e} is the most natural choise. In this case, the increment Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle Q} is equal to the asymtotic value Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle L} .

At the translation for inity along the real axis,

Base e. Superlogarithm

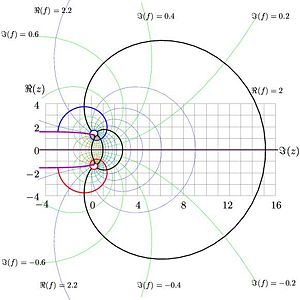
Fig.7. Inverse of the analytic tetration Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=F_{\rm e}^{-1}(z)} at the complex Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z} -plane.

Inverse of the natural tetration can be considered as superlogarithm. Equilines of funciton Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle f=F_{\rm e}^{-1}(z)} are shown in Figure 7. Levels of integer real part and those of integer imaginary part are shown with thick lines. This function has two branchpoints at eigenvalues of logarithm. In the figure, the cuts are placed along the lines Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \Re(f)=-2} ; then, the function is regular in vicinity of the real axis, approaching the limitig value Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -2} at Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle -\infty} and slowly growing up at positive values of the argument.

Discussion

Analytic tetrations are shown for base Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\sqrt{2}} , Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=2} , and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=\rm e} . In the similar way, the tetration on other bases (for example, Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b=10} ) can be plotted.

Knowledge of the asymptotic behavior gives the key to the efficient evaluation.

Conclusions

references

  1. ↑ Publications (Those about tetrations are at the top) http://www.ils.uec.ac.jp/~dima/PAPERS
  2. ↑ Hoos
  3. ↑ All animals are created equal and that with addition BUT SOME ANIMALS ARE MORE EQUAL THAN OTHERS are slogans of animals in the novel Animal farm by George Orwell. (Animals gained the superior power at the farm, bushing out the farmer). First slogan was used to gain the power. The second part appeared when pigs begun to justify their privilegies.

See also the discussion at http://math.eretrandre.org/tetrationforum/forumdisplay.php?fid=3

Table of superfunctions

There exist many basefuncitons such that the supercunctions can be expressed in the closed form. For some function Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F} such that both Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F} and Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F^{-1}} can be expressed with simple and explicit formulas, the basefunciton

.

In such a way, the table below can be constructed.

Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F(z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F^{-1}(z)} comment
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z+\!\!+} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b+z} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z-b}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z+b} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle bz+c} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (z-c)/b}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle cz+c} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (b^z+\frac{c}{1-b})d} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \log_b\frac{z-\frac{cd}{1-b}}{d}}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle b^z} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathrm{tet}_b(z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \mathrm{arctet}_b(z)} tetration
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle z^b} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \exp(b^z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \log_b(\ln(z))}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \big(a^b+z^b\big)^{1/b}} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle az^{1/b}} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle (z/a)^b}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(a+\mathrm{e}^z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \ln(az)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \exp(z)/a}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle az/(z+a)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a/z} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle a/z} amaising,
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle 2z^2-1} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \cos(\pi 2^z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle \log_2(\arccos(z)/\pi)}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle H(z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F(z)} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F^{-1}(z)}
Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P(H(Q(z)))} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle P(F(z))} Failed to parse (SVG (MathML can be enabled via browser plugin): Invalid response ("Math extension cannot connect to Restbase.") from server "https://wikimedia.org/api/rest_v1/":): {\displaystyle F^{-1}(Q(z))} P(Q(z))=z
Retrieved from "https://citizendium.org/wiki/index.php?title=User:Dmitrii_Kouznetsov/Analytic_Tetration&oldid=958302"