James Clerk Maxwell: Difference between revisions
imported>Paul Wormer (→On Faraday's Lines of Force: Adaptation after reading 1911 EB) |
mNo edit summary |
||
| (46 intermediate revisions by 11 users not shown) | |||
| Line 1: | Line 1: | ||
{{subpages}} | {{subpages}} | ||
'''James Clerk Maxwell''' (Edinburgh, June 13, 1831 – Cambridge, November 5, 1879) was a Scottish physicist best known for his formulation of electromagnetic theory and the statistical theory of gases. He is regarded by most modern physicists as the scientist of the 19th century who had the greatest influence on 20th-century physics, and | '''James Clerk Maxwell''' (Edinburgh, June 13, 1831 – Cambridge, November 5, 1879) was a [[Scotland|Scottish]] physicist best known for his formulation of [[electromagnetism|electromagnetic theory]] and the [[kinetic gas theory|statistical theory of gases]]. He also provided quantifiable evidence for the three-colour theory of [[light]] and the correct theory of the [[ring (astronomy)|rings]] of [[Saturn (planet)|Saturn]]. He is regarded by most modern physicists as the scientist of the 19th century who had the greatest influence on 20th-century physics, and is ranked with [[Galileo Galilei]], [[Isaac Newton]], and [[Albert Einstein]], and the main creators of [[quantum mechanics]], [[Werner Heisenberg]], [[Erwin Schrödinger]], and [[Paul A. M. Dirac]]. | ||
The [[Maxwell equations]], the [[Maxwell-Boltzmann distribution]], and the unit of magnetic flux, the [[Maxwell (unit)|maxwell]], were named after him, as was a [[crater]] on the [[moon]]. | |||
[[Image:James Clerk Maxwell.jpg|right|350px]] | [[Image:James Clerk Maxwell.jpg|right|350px]] | ||
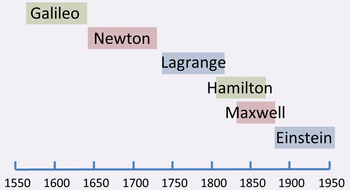
{{Image|Classical mechanics timeline.PNG|right|350px|Timeline for key scientists in classical mechanics}} | |||
==Biography== | ==Biography== | ||
===Family=== | ===Family=== | ||
James Clerk Maxwell came from two prominent and | James Clerk Maxwell came from two prominent and affluent Scottish families, the Maxwells and the Clerks, both of lower nobility and heavily interrelated.<ref>For instance, James's great-grandmother from father's side was Dorothea, Lady Clerk Maxwell. She was the daughter of William and Agnes (née Maxwell) Clerk Maxwell. Dorothea married Sir George Clerk and — as usual in those days — carried the married name Clerk, instead of her father's name Clerk Maxwell.</ref> | ||
After marrying Frances Cay in 1826, | James's father, John, was a younger paternal grandchild of Sir George Clerk, and accordingly was simply named John Clerk, while his elder brother had inherited the title and name of their grandfather, and thus was also called Sir George Clerk. John legally added the name Maxwell later, after he had inherited from his grandmother Dorothea (née Lady Clerk Maxwell) the Middlebie estate in the [[Galloway]] region, in south-west Scotland. The estate is 10 kilometres north of [[Castle Douglas]], the market town, and about 70 kilometres west of the town of [[Middlebie]], Dumfriesshire. | ||
After marrying Frances Cay in 1826, John Clerk Maxwell moved with his bride from [[Edinburgh]] to the Middlebie estate. There they built a country house named Glenlair. The house was in a rather isolated spot, even by Scottish standards, the closest cities being [[Glasgow]] (110 kilometres north, a full day's journey at the time) and Edinburgh, which was two whole days of travel away. | |||
===Early youth=== | ===Early youth=== | ||
James was born in [[Edinburgh]], where his parents had gone to ensure proper medical attention at his birth. | James was born in [[Edinburgh]], where his parents had gone to ensure proper medical attention at his birth. He was the first son of his mother, who earlier had lost a daughter of a few months old. Mrs Clerk Maxwell was at the relatively advanced age of 40 when she gave birth to James. Soon after the birth the family went back to the Glenlair house. James, who remained their only child, was brought up in the Galloway region, where he played with the local children and acquired a broad Scottish accent, despite his upper-class ancestry. | ||
Maxwell's mother died in 1839 from [[abdominal cancer]], the same disease to which Maxwell would succumb at exactly the same age, 48 years. James grew up alone with his father, with whom he had a happy and close relationship. At a young age James received private lessons from a dull and uninspired tutor, who claimed that James was slow at learning, though in fact he was very inquisitive and had a phenomenal memory. At the age of 10 James was sent to the Edinburgh Academy to receive a proper education. During term time, he was living either with his mother's unmarried sister Jane Cay, or with his father's sister Isabella, the widow of James Wedderburn. Both aunts lived in Edinburgh. | |||
At the academy he became a lifelong friend of [[Peter Guthrie Tait]], who would become also a distinguished mathematical physicist and one of the advocates of [[William Rowan Hamilton|Hamilton]]'s [[quaternion]] theory. In the beginning James had a difficult time at school because of his Galloway accent and because he wore strange but practical clothes, designed by his father. | |||
Maxwell was showing unusual mathematical ability at school, and at the age of 15 invented, by analogy with the construction of an ellipse, a way of drawing [[oval]]s using a piece of string. This work was published in the ''Proceedings of the Royal Society of Edinburgh'', and although not an important paper, is remarkable for such a young author. | |||
===Student days=== | ===Student days=== | ||
At the age of | At the age of 16 Maxwell entered [[Edinburgh University]]. He was not unusually young to enter a Scottish university; at that time, these were hybrids between modern secondary schools and universities. Maxwell studied mathematics, philosophy, and physics and left after three years without graduating to go up to [[Cambridge University|Cambridge]], at first to [[Peterhouse College]], which housed many Scotsmen, and after one term to [[Trinity College]]. | ||
By the time he entered | By the time he entered Cambridge, Maxwell was very knowledgeable about [[English literature]] and was a reasonably good [[poet]]. And, of course, he had a vast knowledge of mathematics and physics. In his first year at Cambridge he had to undertake systematic studies, as any student, although he had already published a few papers, including a valuable one on elasticity. His [[tutor]], the mathematician [[William Hopkins]], noted his strong geometrical insight but also that in analysis his powers were somewhat less. Maxwell followed the lectures by [[George Gabriel Stokes|G. G. Stokes]] and encountered [[William Thomson]] again, whom he had first met while at Edinburgh University. | ||
In 1854 Maxwell was second wrangler (second place at the competitive exam the "mathematical tripos") and shared Smith's | In 1854 Maxwell was second wrangler (second place at the competitive exam, the "mathematical tripos", behind [[Edward John Routh]]) and shared the Smith's Prize (a prestigious competitive award for an essay that incorporates original research) with Routh. The following year, Maxwell was elected to a fellowship at his college, Trinity, and started serious research on two of his favourite topics, [[James Clerk Maxwell#Colour Theory|colour theory]] and [[James Clerk Maxwell#Electricity and magnetism|electromagnetism]]. | ||
===Aberdeen=== | ===Aberdeen=== | ||
Clerk Maxwell kept his fellowship at Trinity only | Clerk Maxwell kept his fellowship at Trinity for only a short time. Because his father's health was deteriorating, he sought a professorship in Scotland. He hoped that the long vacations of Scottish universities would permit him to spend many months each year at his beloved Glenlair with his father. In addition, some of Maxwell's letters to his father seem to indicate that the prospect of becoming a Cambridge don did not appeal to him; he recognized the narrowing tendencies of college life. He became Professor of Natural Philosophy at Marischal College, [[Aberdeen]], but before the appointment was announced his father died, in early April 1856. In June 1858, Maxwell married Katherine Mary Dewar, the daughter of the principal of Marischal College and seven years James' senior. The marriage remained childless. | ||
Maxwell's most important work in Aberdeen, besides the continuation of his studies on colour, was a theoretical study on [[James Clerk Maxwell#Saturn's rings|the nature of Saturn's rings]]. He proved that the rings must consist of myriad small particles, or moonlets, each in their own orbit around the planet, and that the ring could neither be solid nor fluid. Maxwell was awarded the £130 [[Adams Prize]] in 1859 for his essay ''On the Stability of Saturn's Rings''. | |||
While in Aberdeen, Maxwell was nominated twice for a Royal Medal of the [[Royal Society]] for his work on the perception of colour, and was twice passed over. But a nomination in May 1860 by G. G. Stokes and [[William Hallowes Miller]] (Cambridge Professor of Mineralogy) for the [[Count Rumford|Rumford Medal]] was successful. | |||
In the fall of 1859, the Chair of Natural Philosophy at Edinburgh became vacant when Maxwell's old teacher and friend [[James D. Forbes]] moved to a less demanding post in [[St Andrews]]. Maxwell and several of his contemporaries applied for this post, including [[Peter Guthrie Tait]] and Edward John Routh. Tait was chosen — he was preferred over Maxwell because it was felt that he was the better teacher. | |||
In 1860 | In 1860 King's College and Marischal College, both in Aberdeen, merged forming the nucleus of what would become [[Aberdeen University]]. Maxwell was made redundant by the merger, even though he was the son-in-law of one of the principals and had won the very prestigious Rumford Medal and the Adams Prize. | ||
After | After having lost his position in Aberdeen and being jobless, Maxwell headed to Glenlair with his wife. He didn't stay unemployed for long and soon found another job. In July 1860, Maxwell was appointed to the vacant Professorship of Natural Philosophy in [[King's College (London)|King's College]], London. Almost immediately after the appointment and before even having moved to London, Maxwell suffered an attack of [[smallpox]]. Because smallpox is very contagious and life-threatening, his servants were afraid to enter the room where Maxwell lay sick, but his wife nursed him lovingly and he soon recovered and started his work at King's college. | ||
===London=== | ===London=== | ||
The work at King's College was more exacting than that in Aberdeen. There were nine months of lecturing in the year, and evening lectures to artisans, | The work at King's College was more exacting than that in Aberdeen. There were nine months of lecturing in the year, and evening lectures to artisans, and so on, were recognised as a part of the professor's regular duties. Maxwell retained the post until the spring of 1865, when he was succeeded by Professor W. G. Adams, but continued lecturing to the artisans during the following winter. In London, Maxwell did most of his great work on electromagnetism, but he also worked on his colour theory and kinetic theory of gases in a laboratory he established in the top floor of his London home. He earned a reputation, at least among his neighbors, as an eccentric scientist. His colour experiments had him gazing into a long, coffin-like, lightbox. His gas experiments were conducted with steam during the dead of summer. His wife, as de-facto lab assistant, kept the stove stoked and water boiling through many hot summer afternoons. Other experiments required cooler temperatures provided by large amounts of ice, probably also hauled to the attic by his wife.<ref>Lewis Campbell and William Garnett (1882) ''The Life of James Clerk Maxwell'' MacMillan, London [http://www.sonnetsoftware.com/bio/maxbio.pdf Online]</ref> These experiments earned him great respect among his peers, as evidenced in June 1861, when Maxwell became, at the age of just 29, a fellow of the Royal Society of London, which entitled him to the [[postnominal]] FRS. | ||
him | |||
Maxwell | |||
===Glenlair=== | ===Glenlair=== | ||
In 1866 | {{Image|Db Glenlair front elevation5.jpg|right|350px|<small>Courtesy Cavendish Laboratory</small><br>Front Glenlair House}} | ||
While out riding—Maxwell was | In 1866 Professor and Mrs. Maxwell returned to Glenlair because of Maxwell's ill health. While out [[riding]] — Maxwell was an enthusiastic horseman — he had grazed his head on a tree branch and the wound had led to a severe attack of [[erysipelas]], an inflammatory disease characterized by severe headaches and vomiting. There is reason to suspect that Maxwell's earlier bout of smallpox had made him sensitive to this disease. | ||
While at Glenlair, he began writing his celebrated two-volume treatise on electricity<ref name = "Treatise">J. Clerk Maxwell (1873) ''A Treatise on Electricity and Magnetism''. Clarendon Press, Oxford. </ref>. It is of interest to note that while Maxwell's original paper of 1865 did not use quaternions — all equations were written out in components — in this work they are used extensively. In his treatise Maxwell refers to a book on quaternions by his friend P. G. Tait.<ref>Tait PG (1985) ''Elementary Treatise on Quaternions'' Oxford</ref> There is evidence<ref>Crowe MJ ''A History of Vector Analysis'' Dover, New York p. 129</ref> that Maxwell familiarized himself with Hamilton's quaternions as late as the early 1870s. Later, [[Maxwell's equations]] were recast by [[Oliver Heaviside]] into the vector format that is still in use today. | |||
During his Glenlair period that lasted until 1871, | During his Glenlair period that lasted until 1871, Maxwell served as examiner and moderator at Cambridge in 1866, 1867, 1869, and 1870, instituting important and useful reforms in the tripos. | ||
Maxwell was a deeply pious man. Lewis Campbell and William Garnett record that visitors to Glenlair between 1866 and 1870 were struck with the manner in which the daily prayers were conducted by the master of the house. The prayers, which seemed extempore, were most impressive and full of meaning. | Maxwell was a deeply [[pious]] man. Lewis Campbell and William Garnett record that visitors to Glenlair between 1866 and 1870 were struck with the manner in which the daily prayers were conducted by the master of the house. The prayers, which seemed extempore, were most impressive and full of meaning.<ref>Lewis Campbell and William Garnett (1882) [http://www.sonnetsoftware.com/bio/maxbio.pdf ''The Life of James Clerk Maxwell''], MacMillan, London </ref> | ||
{{Image|Db Mr and Mrs James Clerk Maxwell5.jpg|left|350px|<small> | |||
Courtesy of the Master and Fellows of Trinity College, University of Cambridge</small><br> | |||
Dr and Mrs James Clerk Maxwell 1869}} | |||
===Back to Cambridge=== | ===Back to Cambridge=== | ||
In 1870 Cambridge University received a splendid gift from William Cavendish, the seventh Duke of Devonshire, who had been second wrangler in 1829 | In 1870 Cambridge University received a splendid gift from William Cavendish, the seventh Duke of Devonshire, who had been second wrangler in 1829 and was in 1870 Chancellor of the university. The duke, directly related to [[Henry Cavendish]], gave the university £6300 for the construction of a physics laboratory. In 1869 a physical sciences syndicate had recommended the foundation of a physical laboratory and the estabishment of a chair in experimental physics. Until then there was no general laboratory in Cambridge; the physics fellows experimented at home or in their colleges. As always, there were insufficient funds to implement the committee's recommendation, which led the duke to intervene and make his splendid offer. The laboratory was to be called the ''[[Cavendish laboratory]]'' and the new chair (£500 per annum) was to be the ''Cavendish Professorship of Experimental Physics''. | ||
William Thomson and [[Hermann von Helmholtz]] were approached for the job, but both declined. Maxwell, at present considered the greatest of the three, was elected in 1871. He thus became the first Cavendish Professor. | |||
Maxwell devoted great energy to the building and equipment of the new laboratory. It was ready for occupation and functioning in 1874 (and would stay in the same place for a full century | Maxwell devoted great energy to the building and equipment of the new laboratory. It was ready for occupation and functioning in 1874 (and would stay in the same place for a full century; the Cavendish moved to a new location in West Cambridge in 1974). Maxwell had started lecturing in October 1871, and in his long inaugural lecture he expressed in detail his philosophy of teaching physics and the function of the laboratory for teaching and research. | ||
During his last Cambridge period, Maxwell undertook the publication of Henry Cavendish's electrical papers. The manuscripts had been gathering dust for about a century. It is believed that they would have influenced greatly the development of electric science had they been known. Cavendish's eccentricity kept them from being published. | During his last Cambridge period, Maxwell undertook the [[scientific publication|publication]] of Henry Cavendish's electrical papers. The manuscripts had been gathering dust for about a century. It is believed that they would have influenced greatly the development of electric science had they been known earlier. Cavendish's eccentricity kept them from being published. | ||
In the summer of 1879, when he was | In the summer of 1879, when he was 48 years old, Maxwell showed signs of the same abdominal cancer that had killed his mother when he was eight. He died on November 5, 1879, the year that his great admirer [[Albert Einstein]] was born. | ||
==Scientific work== | ==Scientific work== | ||
=== | ===Colour Theory=== | ||
Clerk Maxwell started studies on | {{Image|Maxwell.JPG|right|250px| Statue of Maxwell, George Street Edinburgh.}} | ||
Clerk Maxwell started studies on colour vision while still at [[Edinburgh]] under the guidance of Professor [[James David Forbes]]. Fundamental progress on colour theory — a subdiscipline of physiological optics — had been made earlier by [[Thomas Young]], who had arrived at the conception of three fundamental colours: red, green, and blue.<ref>It is interesingt to note in this context that the competing colour theory (''Zur Farbenlehre'') of Young's famous German contemporary, [[Johann Wolfgang von Goethe|Goethe]], has had no impact on scientific colour theory.</ref> Maxwell continued Young's investigations by studying colour perception with a spinning top that allowed quantitative measurement of the colours being mixed. Maxwell's work contributed importantly to the unravelling of the mysteries surrounding colour vision. As a spin-off of his researches, he was able to show the first ever colour [[slide]], which he projected at the [[Royal Institution]] on May 17, 1861.<ref>J. Clerk Maxwell(1861) On the theory of three primary colours'' Proc Royal Institution Great Britain'' 3:370-4 </ref> A hundred years later, it was found<ref>Evans RM (1961) Maxwell’s colour photograph ''Scientific American'' 205:118–27</ref> that Maxwell was lucky that his strategy in designing the slide worked, because ultraviolet acted as a proxy for red in the recording stage, a fact of which Maxwell was unaware. In any case, his slide projection greatly impressed his audience. The work of Young and Maxwell is known today to any computer user who is trying to create his own colours by the [[RGB]] colour scheme.<ref>Dougal RC ''et al.'' (2006) Then and now: James Clerk Maxwell and colour ''Optics Laser Technol''38:210–8. An in-depth review of Maxwell's work on colour theory with a link to present day applications of colour science. http://dx.doi.org/10.1016/j.optlastec.2005.06.036</ref> | |||
===Saturn's rings=== | ===Saturn's rings=== | ||
The planet [[Saturn (planet)|Saturn]] has a flat | The planet [[Saturn (planet)|Saturn]] has a flat circular ring, first observed (but not understood) by [[Galileo Galilei]] in 1610. Dutch physicist [[Christiaan Huygens]] recognized it as a ring in 1655 and 20 years later [[Giovanni Domenico Cassini]] saw that there were actually two rings. In 1850 Harvard astronomer [[George Phillips Bond]] discovered a dark obscure ring interior to these two rings. On a visit to Europe in 1851 Bond spoke about his discovery in Cambridge, where he aroused the interest of [[James Challis]], the [[Plumian chair|Plumian Professor]] of Astronomy. The mechanical stability of the ring was hardly understood at that time. The great [[Laplace]] had studied it and found that a solid uniform ring would be dynamically unstable and could not revolve permanently around the planet. | ||
Challis | Challis set the stability of Saturn's rings as the subject of the 1857 Adams Prize essay. This prize had been established in 1848 by a few members of St John's college of Cambridge University in honour of [[John Couch Adams]]'s prediction of the existence of the planet [[Neptune (planet)|Neptune]]. Only Cambridge graduates could enter the competition. Clerk Maxwell took up the challenge and submitted his essay on December 16, 1856; his was the only submission. | ||
In the first part of his essay he showed that the rings cannot be homogeneously solid, because, in accordance with Laplace's earlier finding, they would be unstable. | In the first part of his essay he showed that the rings cannot be homogeneously solid, because, in accordance with Laplace's earlier finding, they would be unstable. The gravitational gradient arising from the main body of the planet would generate stresses that normal matter could not withstand. | ||
In the second part of his prize essay Maxwell assumed that the rings were fluid (but not at rest because they would then collapse into Saturn). This assumption | In the second part of his prize essay Maxwell assumed that the rings were fluid (but not at rest because they would then collapse into Saturn). This assumption fared no better under realistic conditions because of the development of destabilizing waves. Therefore he was led to assume that the only system of rings that can exist is one composed of an indefinite number of unconnected solid particles, revolving around the planet with different velocities according to their respective distances. He thus concluded that the rings consisted of what were effectively clouds of small solid satellites. This is the correct description, as confirmed by the [[Voyager program|Voyager]] 2 space probe in 1981. | ||
Maxwell was awarded the Adams prize on 30 | Maxwell was awarded the Adams prize on May 30, 1857. Two years later he summarized his more than 80 pages essay in a short article.<ref>Maxwell JC (1859) On the stability of the motion of Saturn's rings'' Monthly Notices, Astronomical Soc London'' 19:297-304 </ref> | ||
===Molecular velocity distribution=== | ===Molecular velocity distribution=== | ||
Kinetic gas theory was established by [[Daniel | [[Kinetic gas theory]] was established by [[Daniel Bernoulli I|Daniel Bernoulli]] in 1738 in a chapter of his book ''Hydrodynamica''. Bernoulli assumed an elastic fluid (i.e., a gas) to consist of "very minute corpuscles, which are driven hither and thither with a very rapid motion".<ref>Newman JR ''The World of Mathematics'', vol. II, p. 774, excerpt from Daniel Bernoulli's book ''Hydrodynamica'' (1738)</ref> Bernoulli was the first to recognize that pressure is caused by collisions of the particles with the walls of the vessel containing the gas, and that the speed of the particles increases with increasing temperature. In the nineteenth century the kinetic theory of gases was pursued, among others, by [[James Prescott Joule]] in England and [[Rudolf Clausius]] in Germany. Especially the latter worker had a determining influence on Maxwell. | ||
Sometime early 1859 Maxwell read a translation of a paper by Clausius, in which the mean path length—the distance covered by | Sometime early 1859 Maxwell read a translation of a paper by Clausius, in which the [[mean path length]]—the distance covered by [[molecule]]s beween successive collisions—played an important role.<ref>Clausius R (1850) On the mean length of the paths described by the separate molecules of gaseous bodies on the occurrence of molecular motion ''Phil Magazine, Ser. 4'' 17:81-91 (1859)</ref> It is likely that Clausius' paper drew Maxwell's attention because he had just finished his work on Saturn's rings in which he had considered the motions and path lengths of myriads of small particles. Maxwell became interested in [[viscosity]], [[diffusion]] rates, and other transport properties of gases. | ||
In his earlier investigations, Clausius assumed that all molecules move with the same speed. He used the mean value of the square of the velocity of molecules as ''the'' speed of the molecule in the gas. Before Maxwell, Clausius never thought of velocity distributions, which express the percentages of | In his earlier investigations, Clausius assumed that all molecules move with the same speed. He used the mean value of the square of the velocity of molecules as ''the'' speed of the molecule in the gas. Before Maxwell, Clausius never thought of velocity [[distribution (statistics)|distributions]], which express the percentages of molecules that have velocities differing from the average velocity. Maxwell, who saw clearly that such statistical distributions could be physically important, wrote a memoir for the British Association in 1859, in which he gave the velocity distribution of the molecules in a gas. He also calculated the [[viscosity coefficient]] of a gas in terms of mean free paths, and wrote about diffusion, and the conduction of heat.<ref>Maxwell JC (1860) ''Illustrations of the dynamical theory of gases I. On the motions and collisons of perfectly elastic spheres ''Phil. Magazine, Ser. 4'' 19:19-32; II. On the process of diffusion of two or more kinds of moving particles among one another'' Phil. Magazine, Ser. 4 '' 20:21-37</ref> | ||
In his kinetic work, Maxwell | In his kinetic work, Maxwell relied heavily on statistical ideas. Maxwell's interest in [[probability theory]] dated back to 1850 when he read an essay by [[John Herschel]] on the work by the Belgian social scientist [[Adolphe Quetelet]], ''Theory of Probabilities''. Later, in 1857, he read the first volume of [[Henry Thomas Buckle]] ''History of Civilization in England'', in which Quetelet's statistical methods are applied to history. It is likely that Maxwell's interests in contemporary debates on statistics led him to applications of probability methods in his 1860 papers on the dynamical theory of gases. The introduction of statistical factors into the treatment of appropriate physical problems must be regarded as one of Maxwell's foremost contributions to the advance of physics. | ||
In his 1860 papers Maxwell used simplified models of a perfect gas in which the principal interaction between molecules occurred during collisions. On the assumption that the three orthogonal components, ''v''<sub>''x''</sub>, ''v''<sub>''y''</sub>, and ''v''<sub>''z''</sub>, of the velocity distribution are independent, he demonstrated that the distribution of velocities, < | In his 1860 papers Maxwell used simplified models of a [[Ideal gas law|perfect gas]] in which the principal interaction between molecules occurred during collisions. On the assumption that the three orthogonal components, ''v''<sub>''x''</sub>, ''v''<sub>''y''</sub>, and ''v''<sub>''z''</sub>, of the velocity distribution are independent, he demonstrated that the distribution of total velocities (speeds), ''v''<sup>2</sup> ≡ ''v''<sub>''x''</sub><sup>2</sup> + ''v''<sub>''y''</sub><sup>2</sup> + ''v''<sub>''z''</sub><sup>2</sup>, among the molecules in the assembly would be governed by a function of the form | ||
:<math> | :<math> | ||
f(v) = A \, v^2 e^{-v^2/B}, | f(v) = A \, v^2 e^{-v^2/B}, | ||
</math> | </math> | ||
in which ''A'' is related to the density of atoms and ''B'' is related to the average kinetic energy of the atoms, or, equivalently, | in which ''A'' is related to the density of atoms and ''B'' is related to the average kinetic energy of the atoms, or, equivalently, the temperature. This function, which is mathematically similar to those that occur in many simple statistical problems, is commonly designated the [[Maxwell distribution law]], or, alternately, the Maxwell-Boltzmann law, in recognition of the work of [[Ludwig Boltzmann]] (1844-1906), who greatly extended research on statistical assemblies. | ||
When Maxwell used his distribution law to calculate the [[viscosity]] of his [[ | When Maxwell used his distribution law to calculate the [[viscosity]] of his [[Ideal gas law|ideal gas]], he was surprised to find that the result was independent of pressure, or density. The same was found to be true of [[thermal conductivity]]. Careful measurements of the viscosity and thermal conductivity of several gases, carried out at home with the aid of his wife, confirmed the theoretical results for appropriate lower ranges of pressure. This success demonstrated that the theory could be used for other calculations. It also provided a basis for estimating atomic size and an accurate determination of [[Avogadro's number]]. | ||
Clausius pointed out some errors in Maxwell's 1860 treatment of diffusion and conduction of heat, which Maxwell corrected in 1866.<ref>J. Clerk Maxwell, ''On the dynamical Theory of Gases'', read May 31, 1866; published in Philosophical Transactions | Clausius pointed out some errors in Maxwell's 1860 treatment of diffusion and conduction of heat, which Maxwell corrected in 1866.<ref>J. Clerk Maxwell, ''On the dynamical Theory of Gases'', read May 31, 1866; published in Philosophical Transactions of the Royal Society of London, vol. '''157''', pp. 49-88 (1867)</ref>. In the same work Maxwell modified his theory in consequence of the results of his experiments on the viscosity of air at different temperatures. Further he introduced an intermolecular repulsive force depending on intermolecular distance ''R'' as ''R''<sup>−5</sup>. | ||
===Maxwell's demon=== | ===Maxwell's demon=== | ||
In 1868 Maxwell mentioned in a letter to his friend Tait a finite being ("Maxwell's demon")<ref>J. Clerk Maxwell, ''Theory of Heat'', Longmans, Green, London (1871); reprinted Dover, New York (2001). Maxwell wrote about a "finite being", the name "demon" was coined by W. Thomson in 1874.</ref> | In 1868 Maxwell mentioned in a letter to his friend Tait a finite being ("Maxwell's demon")<ref>J. Clerk Maxwell, ''Theory of Heat'', Longmans, Green, London (1871); reprinted Dover, New York (2001). Maxwell wrote about a "finite being", the name "demon" was coined by W. Thomson in 1874.</ref> that could violate the [[Laws of thermodynamics|second law of thermodynamics]]. This law had been formulated in the 1850s by [[William Thomson]] and [[Rudolf Clausius]]. Clausius stated that it is impossible that heat flows spontaneously up a temperature slope, i.e., heat does not go naturally from a low temperature bath to one of higher temperature; work is required to achieve such a transport of energy. | ||
Maxwell thought up a vessel, filled with an ideal gas, that is divided into two partitions ''A'' and ''B'' by a division in which there is a small hole. The hole is guarded by a being, ''Maxwell's demon'', who can see the individual molecules and who opens and closes the hole, so as to allow only the swifter molecules to pass from ''A'' to ''B'' | Maxwell thought up a vessel, filled with an ideal gas, that is divided into two partitions ''A'' and ''B'' by a division in which there is a small hole. The hole is guarded by a being, ''Maxwell's demon'', who can see the individual molecules and who opens and closes the hole, so as to allow only the swifter molecules to pass from ''A'' to ''B'' and only the slower ones to pass from ''B'' to ''A''. He or she will, thus, without performing any work raise the temperature of ''B'' and lower that of ''A''. The internal energy of an ideal gas being proportional to temperature, the demon decreases the energy of ''A'' and increases that of ''B''. In other words, the demon causes heat to flow from the low temperature partition (''A'') to the high temperature partition (''B'') without expenditure of work, in contradiction to Clausius' expression of the second law. Since this process is the opposite of a natural (spontaneous) process in which [[entropy]] increases, it is clear that the entropy of the total system ''A'' + ''B'' decreases by the action of Maxwell's demon.<ref>One can confirm this easily for a monoatomic ideal gas by direct calculation. The energy of one mole of such gas is 3''RT''/2, with ''R'' the [[molar gas constant]] and ''T'' the absolute [[temperature]]. The entropy of one mole is ''S''(''T'') = ''R'' ln(''V''•''T''<sup> 3/2</sup>) + ''S''(0). Assume that the amount of gas in ''A'' and ''B'' stays constant (one mole each) during the demon's operation and that both partitions have the same volume ''V''. By conservation of energy | ||
:<math>\scriptstyle | :<math>\scriptstyle | ||
\Delta T^A + \Delta T^B =\, 0. | \Delta T^A + \Delta T^B =\, 0. | ||
</math> | </math> | ||
Assume further that the initial temperature of ''A'' and ''B'' is equal to ''T''. Under these conditions the entropy change, caused by the demon, is | Assume further that the initial temperature of ''A'' and ''B'' is equal to ''T''. Under these conditions the entropy change, caused by the demon, is | ||
:<math>\scriptstyle | :<math>\scriptstyle | ||
\Delta S^A + \Delta S^B = \frac{3}{2}\,R\, \ln\Big[1 - \left(\frac{\Delta T^A}{T}\right)^2\Big]. | \Delta S^A + \Delta S^B = \frac{3}{2}\,R\, \ln\Big[1 - \left(\frac{\Delta T^A}{T}\right)^2\Big]. | ||
</math> | </math> | ||
Because the temperature decrease Δ''T''<sup>''A''</sup> is limited by ''T''<sup>''A''</sup> | Because the temperature decrease Δ''T''<sup>''A''</sup> is limited by ''T''<sup>''A''</sup> = 0 and ''T''<sup>''A''</sup> = ''T'', it follows that the argument of the logarithm lies between zero and unity, and hence the logarithm is negative and so is the entropy change of ''A'' + ''B''. </ref> | ||
Much has been written about a solution of this paradox. A simple-minded answer is that, according to quantum mechanics, individual molecules are indistinguishable, so that the demon cannot see individual molecules. A different solution was discussed by [[Leo Szilard]] in 1929,<ref> | Much has been written about a solution of this paradox. A simple-minded answer is that, according to quantum mechanics, individual molecules are indistinguishable, so that the demon cannot see individual molecules. A different solution was discussed by [[Leo Szilard]] in 1929,<ref> | ||
L. Szilard, ''Über die Entropieverminderung in einem thermodynamischen System bei Eingriffen intelligenter Wesen.'' [On the decrease of entropy in a thermodynamic system | L. Szilard, ''Über die Entropieverminderung in einem thermodynamischen System bei Eingriffen intelligenter Wesen.'' [On the decrease of entropy in a thermodynamic system | ||
by the intervention of intelligent beings]. Zeitschrift für Physik, vol. '''53''', pp. 840-856 (1929) [http://dx.doi.org/10.1007/BF01341281 doi]</ref> who argued that the demon is part of the total system and that the demon's [[entropy]] increases by receiving and storing information. The demon's increase in entropy must be added to the total entropy and thus cancels the decrease in entropy of the gas in the two chambers. For refinements of Szilard's argument, other solutions, and the relation of entropy to [[information theory]], see Ref.<ref>''Maxwell's Demon 2, Entropy, Classical and Quantum Information, Computing'' edited by Harvey S. Leff and Andrew F. Rex, Institute of Physics Publishing, Bristol, 2nd edition, 2003 </ref> | by the intervention of intelligent beings]. Zeitschrift für Physik, vol. '''53''', pp. 840-856 (1929) [http://dx.doi.org/10.1007/BF01341281 doi]</ref> who argued that the demon is part of the total system and that the demon's [[entropy]] increases by receiving and storing information. The demon's increase in entropy must be added to the total entropy and thus cancels the decrease in entropy of the gas in the two chambers. For refinements of Szilard's argument, other solutions, and the relation of entropy to [[information theory]], see Ref.<ref>''Maxwell's Demon 2, Entropy, Classical and Quantum Information, Computing'' edited by Harvey S. Leff and Andrew F. Rex, Institute of Physics Publishing, Bristol, 2nd edition, 2003 </ref>; a web source, describing the paradox and its solutions, is in Ref.<ref> [http://splasho.com/blog/maxwell-thermodynamics-meets-the-demon/ Maxwell's demon] - blog post on the history of thermodynamics, with detailed treatment of the demon</ref>. | ||
===Electricity and magnetism=== | ===Electricity and magnetism=== | ||
James Clerk Maxwell's derivation of the classical field equations of electromagnetism represents his greatest triumph. | James Clerk Maxwell's derivation of the classical field equations of electromagnetism represents his greatest triumph. This accomplishment places him in the rank of the very great physicists, such as Galileo, Newton, and Einstein. | ||
====On Faraday's Lines of Force ==== | ====On Faraday's Lines of Force ==== | ||
As an undergraduate in Cambridge University | As an undergraduate in Cambridge University, Maxwell read [[Michael Faraday]]'s ''Experimental Researches in Electricity'' (1839-1855), in which Faraday explained magnetism by [[lines of force|force lines]] traversing space, instead of by actions at a distance. Thomson had cast some of Faraday's ideas into mathematical form and guided by his work Maxwell went a step further. In his first paper on electromagnetism (1855), entitled ''On Faraday's Lines of Force'',<ref>J. Clerk Maxwell, ''On Faraday's Lines of Force'', Trans. Cambridge Philosophical Society, vol. '''10''', pp. 27-83 (1855)</ref> one of his aims was to overturn the idea of action at a distance.<ref>P. G. Tait in a posthumous article on Maxwell in the 1911 edition of the Encyclopaedia Britannica. [http://www.1911encyclopedia.org/James_Clerk_Maxwell Online]</ref> | ||
article on Maxwell in 1911 | |||
Maxwell presented a—what he called—geometrical model of lines of force in space. This representation rested on equipotential | Maxwell presented a — what he called — geometrical model of lines of force in space. This representation rested on [[equipotential surface]]s (scalar functions) to which the force lines (gradient vectors) are orthogonal and give paths of steepest descent from one equipotential surface to the next. Maxwell was guided by the physical analogy of incompressible fluids; not that Maxwell believed electricity to be a fluid, but he recognized the mathematical analogy of field lines to the velocity vectors of flowing fluids. | ||
====On Physical Lines of Force==== | ====On Physical Lines of Force==== | ||
Five years later, in 1861, Maxwell returned to the subject and wrote a series of four papers entitled ''On Physical Lines of Force''.<ref>J. Clerk Maxwell, ''On Physical Lines of Force'', Philosophical Magazine 4th series, Four Parts, I: vol. '''11''', pp. 161-175; II: vol. '''11''', pp. 281-291, 338-347; III: vol. '''13''', pp. 12-23; IV: vol. '''13''', pp. 85-95 (1861, 1862).[http://upload.wikimedia.org/wikipedia/commons/b/b8/On_Physical_Lines_of_Force.pdf online]</ref> | Five years later, in 1861, Maxwell returned to the subject and wrote a series of four papers entitled ''On Physical Lines of Force''.<ref>J. Clerk Maxwell, ''On Physical Lines of Force'', Philosophical Magazine 4th series, Four Parts, I: vol. '''11''', pp. 161-175; II: vol. '''11''', pp. 281-291, 338-347; III: vol. '''13''', pp. 12-23; IV: vol. '''13''', pp. 85-95 (1861, 1862).[http://upload.wikimedia.org/wikipedia/commons/b/b8/On_Physical_Lines_of_Force.pdf online]</ref> In this work he introduced a mechanical [[ether (physics)|ether]] model, based on "molecular vortices" and "idle wheel particles", that is rather foreign to the modern reader. However, he inferred two momentous consequences: the usual conduction current has to be augmented with a [[displacement current]] and the ether sustains transversal vibrations propagating with a velocity ''c'', now known as the [[speed of light]]. | ||
To put the second consequence into perspective it must be recalled that in 1856 | To put the second consequence into perspective it must be recalled that in 1856 ''c'' had been measured by [[Wilhelm Eduard Weber]] and [[Rudolf Kohlrausch]] as the factor connecting two different definitions of electric charge (see [[statcoulomb]] and [[abcoulomb]]): by [[Coulomb's law]] and by [[Ampère's equation]]. Almost immediately [[Gustav Kirchhoff]] noticed that their value of ''c'' was surprisingly close to the speed of light determined by [[Hippolyte Louis Fizeau]] in 1849. And now Maxwell had shown that [[electromagnetic wave]]s propagate with exactly the same speed. | ||
In the introduction of part III of his ''On Physical Lines of Force'' Maxwell puts forward the supposition | In the introduction of part III of his ''On Physical Lines of Force'' Maxwell puts forward the supposition that there is only one medium, one ether, carrying both light and electromagnetic phenomena. On p. 22 of the same paper, he writes the following paragraph which is one of the landmarks in the history of the exact sciences: | ||
<blockquote> | <blockquote> | ||
''The velocity of transverse undulations in our hypothetical medium, calculated from the electro-magnetic experiments of MM. Kohlrausch and Weber, agrees so exactly with the velocity of light calculated from the optical experiments of M. Fizeau, that we can scarcely avoid the inference that light consists in the transverse undulations of the same medium which is the cause of electric and magnetic phenomena.'' | ''The velocity of transverse undulations in our hypothetical medium, calculated from the electro-magnetic experiments of MM. Kohlrausch and Weber, agrees so exactly with the velocity of light calculated from the optical experiments of M. Fizeau, that we can scarcely avoid the inference that light consists in the transverse undulations of the same medium which is the cause of electric and magnetic phenomena.'' | ||
</blockquote> | </blockquote> | ||
====A Dynamical Theory of the Electromagnetic Field==== | ====A Dynamical Theory of the Electromagnetic Field==== | ||
In 1865 Maxwell wrote his most important paper | In 1865 Maxwell wrote his most important paper "A Dynamical Theory of the Electromagnetic Field'',<ref>J. Clerk Maxwell, ''A Dynamical Theory of the Electromagnetic Field" ''Phil. Trans. Roy. Soc'' 155:459 - 512 (1865) [http://upload.wikimedia.org/wikipedia/commons/1/19/A_Dynamical_Theory_of_the_Electromagnetic_Field.pdf online]) </ref> in which he introduces a set of equations, now known as the [[Maxwell equations]]. This paper is very accessible, also by the modern reader, because he abandoned his mechanical model of vortices and idle wheels, and only applies concepts that are still being taught in modern university courses. | ||
He presents twenty equations, | He presents twenty equations, divided in eight groups labeled (A) to (H), with twenty variables. | ||
Since he did not yet know vector or quaternion notation the different components of his [[vector field]]s are counted as individual parameters and the equations are written out in components. | Since he did not yet know vector or quaternion notation the different components of his [[vector field]]s are counted as individual parameters and the equations are written out in components. For a representation of his equations in modern vector form and comments on them, see the ''[[James Clerk Maxwell/Addendum|Addendum]]''. | ||
====The Treatise==== | ====The Treatise==== | ||
Maxwell's electrical work was ultimately summed up in his ''Treatise on Electricity and Magnetism''<ref name = "Treatise"/>. The first edition appeared in 1873, the second in 1881, after the author's death, and was edited by [[W. D. Niven]], although it had been revised in part by Maxwell. The third edition, 1891, was edited by [[Joseph John Thomson]]. The book is not a systematic treatise in the ordinary sense, and it would be hard to go through it from cover to cover. | Maxwell's electrical work was ultimately summed up in his ''Treatise on Electricity and Magnetism''<ref name = "Treatise"/>. The first edition appeared in 1873, the second in 1881, after the author's death, and was edited by [[W. D. Niven]], although it had been revised in part by Maxwell. The third edition, 1891, was edited by [[Joseph John Thomson]]. The book is not a systematic [[treatise]] in the ordinary sense, and it would be hard to go through it from cover to cover. It contains lots of interesting material and insights, but this has to be dug out the hard way. Maxwell's equations appear only in Chapter IX of part 4, well into the second half of the second volume. Maxwell uses both component and quaternion notation. The book appeared to contemporary physicists, and even to the immediate following generations, as a great but inaccessible monument. | ||
==References and notes== | ==References and notes== | ||
{{reflist|2}}[[Category:Suggestion Bot Tag]] | |||
Latest revision as of 16:01, 3 September 2024
James Clerk Maxwell (Edinburgh, June 13, 1831 – Cambridge, November 5, 1879) was a Scottish physicist best known for his formulation of electromagnetic theory and the statistical theory of gases. He also provided quantifiable evidence for the three-colour theory of light and the correct theory of the rings of Saturn. He is regarded by most modern physicists as the scientist of the 19th century who had the greatest influence on 20th-century physics, and is ranked with Galileo Galilei, Isaac Newton, and Albert Einstein, and the main creators of quantum mechanics, Werner Heisenberg, Erwin Schrödinger, and Paul A. M. Dirac.
The Maxwell equations, the Maxwell-Boltzmann distribution, and the unit of magnetic flux, the maxwell, were named after him, as was a crater on the moon.
Biography
Family
James Clerk Maxwell came from two prominent and affluent Scottish families, the Maxwells and the Clerks, both of lower nobility and heavily interrelated.[1]
James's father, John, was a younger paternal grandchild of Sir George Clerk, and accordingly was simply named John Clerk, while his elder brother had inherited the title and name of their grandfather, and thus was also called Sir George Clerk. John legally added the name Maxwell later, after he had inherited from his grandmother Dorothea (née Lady Clerk Maxwell) the Middlebie estate in the Galloway region, in south-west Scotland. The estate is 10 kilometres north of Castle Douglas, the market town, and about 70 kilometres west of the town of Middlebie, Dumfriesshire.
After marrying Frances Cay in 1826, John Clerk Maxwell moved with his bride from Edinburgh to the Middlebie estate. There they built a country house named Glenlair. The house was in a rather isolated spot, even by Scottish standards, the closest cities being Glasgow (110 kilometres north, a full day's journey at the time) and Edinburgh, which was two whole days of travel away.
Early youth
James was born in Edinburgh, where his parents had gone to ensure proper medical attention at his birth. He was the first son of his mother, who earlier had lost a daughter of a few months old. Mrs Clerk Maxwell was at the relatively advanced age of 40 when she gave birth to James. Soon after the birth the family went back to the Glenlair house. James, who remained their only child, was brought up in the Galloway region, where he played with the local children and acquired a broad Scottish accent, despite his upper-class ancestry.
Maxwell's mother died in 1839 from abdominal cancer, the same disease to which Maxwell would succumb at exactly the same age, 48 years. James grew up alone with his father, with whom he had a happy and close relationship. At a young age James received private lessons from a dull and uninspired tutor, who claimed that James was slow at learning, though in fact he was very inquisitive and had a phenomenal memory. At the age of 10 James was sent to the Edinburgh Academy to receive a proper education. During term time, he was living either with his mother's unmarried sister Jane Cay, or with his father's sister Isabella, the widow of James Wedderburn. Both aunts lived in Edinburgh.
At the academy he became a lifelong friend of Peter Guthrie Tait, who would become also a distinguished mathematical physicist and one of the advocates of Hamilton's quaternion theory. In the beginning James had a difficult time at school because of his Galloway accent and because he wore strange but practical clothes, designed by his father.
Maxwell was showing unusual mathematical ability at school, and at the age of 15 invented, by analogy with the construction of an ellipse, a way of drawing ovals using a piece of string. This work was published in the Proceedings of the Royal Society of Edinburgh, and although not an important paper, is remarkable for such a young author.
Student days
At the age of 16 Maxwell entered Edinburgh University. He was not unusually young to enter a Scottish university; at that time, these were hybrids between modern secondary schools and universities. Maxwell studied mathematics, philosophy, and physics and left after three years without graduating to go up to Cambridge, at first to Peterhouse College, which housed many Scotsmen, and after one term to Trinity College.
By the time he entered Cambridge, Maxwell was very knowledgeable about English literature and was a reasonably good poet. And, of course, he had a vast knowledge of mathematics and physics. In his first year at Cambridge he had to undertake systematic studies, as any student, although he had already published a few papers, including a valuable one on elasticity. His tutor, the mathematician William Hopkins, noted his strong geometrical insight but also that in analysis his powers were somewhat less. Maxwell followed the lectures by G. G. Stokes and encountered William Thomson again, whom he had first met while at Edinburgh University.
In 1854 Maxwell was second wrangler (second place at the competitive exam, the "mathematical tripos", behind Edward John Routh) and shared the Smith's Prize (a prestigious competitive award for an essay that incorporates original research) with Routh. The following year, Maxwell was elected to a fellowship at his college, Trinity, and started serious research on two of his favourite topics, colour theory and electromagnetism.
Aberdeen
Clerk Maxwell kept his fellowship at Trinity for only a short time. Because his father's health was deteriorating, he sought a professorship in Scotland. He hoped that the long vacations of Scottish universities would permit him to spend many months each year at his beloved Glenlair with his father. In addition, some of Maxwell's letters to his father seem to indicate that the prospect of becoming a Cambridge don did not appeal to him; he recognized the narrowing tendencies of college life. He became Professor of Natural Philosophy at Marischal College, Aberdeen, but before the appointment was announced his father died, in early April 1856. In June 1858, Maxwell married Katherine Mary Dewar, the daughter of the principal of Marischal College and seven years James' senior. The marriage remained childless.
Maxwell's most important work in Aberdeen, besides the continuation of his studies on colour, was a theoretical study on the nature of Saturn's rings. He proved that the rings must consist of myriad small particles, or moonlets, each in their own orbit around the planet, and that the ring could neither be solid nor fluid. Maxwell was awarded the £130 Adams Prize in 1859 for his essay On the Stability of Saturn's Rings.
While in Aberdeen, Maxwell was nominated twice for a Royal Medal of the Royal Society for his work on the perception of colour, and was twice passed over. But a nomination in May 1860 by G. G. Stokes and William Hallowes Miller (Cambridge Professor of Mineralogy) for the Rumford Medal was successful.
In the fall of 1859, the Chair of Natural Philosophy at Edinburgh became vacant when Maxwell's old teacher and friend James D. Forbes moved to a less demanding post in St Andrews. Maxwell and several of his contemporaries applied for this post, including Peter Guthrie Tait and Edward John Routh. Tait was chosen — he was preferred over Maxwell because it was felt that he was the better teacher.
In 1860 King's College and Marischal College, both in Aberdeen, merged forming the nucleus of what would become Aberdeen University. Maxwell was made redundant by the merger, even though he was the son-in-law of one of the principals and had won the very prestigious Rumford Medal and the Adams Prize.
After having lost his position in Aberdeen and being jobless, Maxwell headed to Glenlair with his wife. He didn't stay unemployed for long and soon found another job. In July 1860, Maxwell was appointed to the vacant Professorship of Natural Philosophy in King's College, London. Almost immediately after the appointment and before even having moved to London, Maxwell suffered an attack of smallpox. Because smallpox is very contagious and life-threatening, his servants were afraid to enter the room where Maxwell lay sick, but his wife nursed him lovingly and he soon recovered and started his work at King's college.
London
The work at King's College was more exacting than that in Aberdeen. There were nine months of lecturing in the year, and evening lectures to artisans, and so on, were recognised as a part of the professor's regular duties. Maxwell retained the post until the spring of 1865, when he was succeeded by Professor W. G. Adams, but continued lecturing to the artisans during the following winter. In London, Maxwell did most of his great work on electromagnetism, but he also worked on his colour theory and kinetic theory of gases in a laboratory he established in the top floor of his London home. He earned a reputation, at least among his neighbors, as an eccentric scientist. His colour experiments had him gazing into a long, coffin-like, lightbox. His gas experiments were conducted with steam during the dead of summer. His wife, as de-facto lab assistant, kept the stove stoked and water boiling through many hot summer afternoons. Other experiments required cooler temperatures provided by large amounts of ice, probably also hauled to the attic by his wife.[2] These experiments earned him great respect among his peers, as evidenced in June 1861, when Maxwell became, at the age of just 29, a fellow of the Royal Society of London, which entitled him to the postnominal FRS.
Glenlair
In 1866 Professor and Mrs. Maxwell returned to Glenlair because of Maxwell's ill health. While out riding — Maxwell was an enthusiastic horseman — he had grazed his head on a tree branch and the wound had led to a severe attack of erysipelas, an inflammatory disease characterized by severe headaches and vomiting. There is reason to suspect that Maxwell's earlier bout of smallpox had made him sensitive to this disease.
While at Glenlair, he began writing his celebrated two-volume treatise on electricity[3]. It is of interest to note that while Maxwell's original paper of 1865 did not use quaternions — all equations were written out in components — in this work they are used extensively. In his treatise Maxwell refers to a book on quaternions by his friend P. G. Tait.[4] There is evidence[5] that Maxwell familiarized himself with Hamilton's quaternions as late as the early 1870s. Later, Maxwell's equations were recast by Oliver Heaviside into the vector format that is still in use today.
During his Glenlair period that lasted until 1871, Maxwell served as examiner and moderator at Cambridge in 1866, 1867, 1869, and 1870, instituting important and useful reforms in the tripos.
Maxwell was a deeply pious man. Lewis Campbell and William Garnett record that visitors to Glenlair between 1866 and 1870 were struck with the manner in which the daily prayers were conducted by the master of the house. The prayers, which seemed extempore, were most impressive and full of meaning.[6]
Back to Cambridge
In 1870 Cambridge University received a splendid gift from William Cavendish, the seventh Duke of Devonshire, who had been second wrangler in 1829 and was in 1870 Chancellor of the university. The duke, directly related to Henry Cavendish, gave the university £6300 for the construction of a physics laboratory. In 1869 a physical sciences syndicate had recommended the foundation of a physical laboratory and the estabishment of a chair in experimental physics. Until then there was no general laboratory in Cambridge; the physics fellows experimented at home or in their colleges. As always, there were insufficient funds to implement the committee's recommendation, which led the duke to intervene and make his splendid offer. The laboratory was to be called the Cavendish laboratory and the new chair (£500 per annum) was to be the Cavendish Professorship of Experimental Physics.
William Thomson and Hermann von Helmholtz were approached for the job, but both declined. Maxwell, at present considered the greatest of the three, was elected in 1871. He thus became the first Cavendish Professor.
Maxwell devoted great energy to the building and equipment of the new laboratory. It was ready for occupation and functioning in 1874 (and would stay in the same place for a full century; the Cavendish moved to a new location in West Cambridge in 1974). Maxwell had started lecturing in October 1871, and in his long inaugural lecture he expressed in detail his philosophy of teaching physics and the function of the laboratory for teaching and research.
During his last Cambridge period, Maxwell undertook the publication of Henry Cavendish's electrical papers. The manuscripts had been gathering dust for about a century. It is believed that they would have influenced greatly the development of electric science had they been known earlier. Cavendish's eccentricity kept them from being published.
In the summer of 1879, when he was 48 years old, Maxwell showed signs of the same abdominal cancer that had killed his mother when he was eight. He died on November 5, 1879, the year that his great admirer Albert Einstein was born.
Scientific work
Colour Theory
Clerk Maxwell started studies on colour vision while still at Edinburgh under the guidance of Professor James David Forbes. Fundamental progress on colour theory — a subdiscipline of physiological optics — had been made earlier by Thomas Young, who had arrived at the conception of three fundamental colours: red, green, and blue.[7] Maxwell continued Young's investigations by studying colour perception with a spinning top that allowed quantitative measurement of the colours being mixed. Maxwell's work contributed importantly to the unravelling of the mysteries surrounding colour vision. As a spin-off of his researches, he was able to show the first ever colour slide, which he projected at the Royal Institution on May 17, 1861.[8] A hundred years later, it was found[9] that Maxwell was lucky that his strategy in designing the slide worked, because ultraviolet acted as a proxy for red in the recording stage, a fact of which Maxwell was unaware. In any case, his slide projection greatly impressed his audience. The work of Young and Maxwell is known today to any computer user who is trying to create his own colours by the RGB colour scheme.[10]
Saturn's rings
The planet Saturn has a flat circular ring, first observed (but not understood) by Galileo Galilei in 1610. Dutch physicist Christiaan Huygens recognized it as a ring in 1655 and 20 years later Giovanni Domenico Cassini saw that there were actually two rings. In 1850 Harvard astronomer George Phillips Bond discovered a dark obscure ring interior to these two rings. On a visit to Europe in 1851 Bond spoke about his discovery in Cambridge, where he aroused the interest of James Challis, the Plumian Professor of Astronomy. The mechanical stability of the ring was hardly understood at that time. The great Laplace had studied it and found that a solid uniform ring would be dynamically unstable and could not revolve permanently around the planet.
Challis set the stability of Saturn's rings as the subject of the 1857 Adams Prize essay. This prize had been established in 1848 by a few members of St John's college of Cambridge University in honour of John Couch Adams's prediction of the existence of the planet Neptune. Only Cambridge graduates could enter the competition. Clerk Maxwell took up the challenge and submitted his essay on December 16, 1856; his was the only submission.
In the first part of his essay he showed that the rings cannot be homogeneously solid, because, in accordance with Laplace's earlier finding, they would be unstable. The gravitational gradient arising from the main body of the planet would generate stresses that normal matter could not withstand.
In the second part of his prize essay Maxwell assumed that the rings were fluid (but not at rest because they would then collapse into Saturn). This assumption fared no better under realistic conditions because of the development of destabilizing waves. Therefore he was led to assume that the only system of rings that can exist is one composed of an indefinite number of unconnected solid particles, revolving around the planet with different velocities according to their respective distances. He thus concluded that the rings consisted of what were effectively clouds of small solid satellites. This is the correct description, as confirmed by the Voyager 2 space probe in 1981.
Maxwell was awarded the Adams prize on May 30, 1857. Two years later he summarized his more than 80 pages essay in a short article.[11]
Molecular velocity distribution
Kinetic gas theory was established by Daniel Bernoulli in 1738 in a chapter of his book Hydrodynamica. Bernoulli assumed an elastic fluid (i.e., a gas) to consist of "very minute corpuscles, which are driven hither and thither with a very rapid motion".[12] Bernoulli was the first to recognize that pressure is caused by collisions of the particles with the walls of the vessel containing the gas, and that the speed of the particles increases with increasing temperature. In the nineteenth century the kinetic theory of gases was pursued, among others, by James Prescott Joule in England and Rudolf Clausius in Germany. Especially the latter worker had a determining influence on Maxwell.
Sometime early 1859 Maxwell read a translation of a paper by Clausius, in which the mean path length—the distance covered by molecules beween successive collisions—played an important role.[13] It is likely that Clausius' paper drew Maxwell's attention because he had just finished his work on Saturn's rings in which he had considered the motions and path lengths of myriads of small particles. Maxwell became interested in viscosity, diffusion rates, and other transport properties of gases.
In his earlier investigations, Clausius assumed that all molecules move with the same speed. He used the mean value of the square of the velocity of molecules as the speed of the molecule in the gas. Before Maxwell, Clausius never thought of velocity distributions, which express the percentages of molecules that have velocities differing from the average velocity. Maxwell, who saw clearly that such statistical distributions could be physically important, wrote a memoir for the British Association in 1859, in which he gave the velocity distribution of the molecules in a gas. He also calculated the viscosity coefficient of a gas in terms of mean free paths, and wrote about diffusion, and the conduction of heat.[14]
In his kinetic work, Maxwell relied heavily on statistical ideas. Maxwell's interest in probability theory dated back to 1850 when he read an essay by John Herschel on the work by the Belgian social scientist Adolphe Quetelet, Theory of Probabilities. Later, in 1857, he read the first volume of Henry Thomas Buckle History of Civilization in England, in which Quetelet's statistical methods are applied to history. It is likely that Maxwell's interests in contemporary debates on statistics led him to applications of probability methods in his 1860 papers on the dynamical theory of gases. The introduction of statistical factors into the treatment of appropriate physical problems must be regarded as one of Maxwell's foremost contributions to the advance of physics.
In his 1860 papers Maxwell used simplified models of a perfect gas in which the principal interaction between molecules occurred during collisions. On the assumption that the three orthogonal components, vx, vy, and vz, of the velocity distribution are independent, he demonstrated that the distribution of total velocities (speeds), v2 ≡ vx2 + vy2 + vz2, among the molecules in the assembly would be governed by a function of the form
in which A is related to the density of atoms and B is related to the average kinetic energy of the atoms, or, equivalently, the temperature. This function, which is mathematically similar to those that occur in many simple statistical problems, is commonly designated the Maxwell distribution law, or, alternately, the Maxwell-Boltzmann law, in recognition of the work of Ludwig Boltzmann (1844-1906), who greatly extended research on statistical assemblies.
When Maxwell used his distribution law to calculate the viscosity of his ideal gas, he was surprised to find that the result was independent of pressure, or density. The same was found to be true of thermal conductivity. Careful measurements of the viscosity and thermal conductivity of several gases, carried out at home with the aid of his wife, confirmed the theoretical results for appropriate lower ranges of pressure. This success demonstrated that the theory could be used for other calculations. It also provided a basis for estimating atomic size and an accurate determination of Avogadro's number.
Clausius pointed out some errors in Maxwell's 1860 treatment of diffusion and conduction of heat, which Maxwell corrected in 1866.[15]. In the same work Maxwell modified his theory in consequence of the results of his experiments on the viscosity of air at different temperatures. Further he introduced an intermolecular repulsive force depending on intermolecular distance R as R−5.
Maxwell's demon
In 1868 Maxwell mentioned in a letter to his friend Tait a finite being ("Maxwell's demon")[16] that could violate the second law of thermodynamics. This law had been formulated in the 1850s by William Thomson and Rudolf Clausius. Clausius stated that it is impossible that heat flows spontaneously up a temperature slope, i.e., heat does not go naturally from a low temperature bath to one of higher temperature; work is required to achieve such a transport of energy.
Maxwell thought up a vessel, filled with an ideal gas, that is divided into two partitions A and B by a division in which there is a small hole. The hole is guarded by a being, Maxwell's demon, who can see the individual molecules and who opens and closes the hole, so as to allow only the swifter molecules to pass from A to B and only the slower ones to pass from B to A. He or she will, thus, without performing any work raise the temperature of B and lower that of A. The internal energy of an ideal gas being proportional to temperature, the demon decreases the energy of A and increases that of B. In other words, the demon causes heat to flow from the low temperature partition (A) to the high temperature partition (B) without expenditure of work, in contradiction to Clausius' expression of the second law. Since this process is the opposite of a natural (spontaneous) process in which entropy increases, it is clear that the entropy of the total system A + B decreases by the action of Maxwell's demon.[17]
Much has been written about a solution of this paradox. A simple-minded answer is that, according to quantum mechanics, individual molecules are indistinguishable, so that the demon cannot see individual molecules. A different solution was discussed by Leo Szilard in 1929,[18] who argued that the demon is part of the total system and that the demon's entropy increases by receiving and storing information. The demon's increase in entropy must be added to the total entropy and thus cancels the decrease in entropy of the gas in the two chambers. For refinements of Szilard's argument, other solutions, and the relation of entropy to information theory, see Ref.[19]; a web source, describing the paradox and its solutions, is in Ref.[20].
Electricity and magnetism
James Clerk Maxwell's derivation of the classical field equations of electromagnetism represents his greatest triumph. This accomplishment places him in the rank of the very great physicists, such as Galileo, Newton, and Einstein.
On Faraday's Lines of Force
As an undergraduate in Cambridge University, Maxwell read Michael Faraday's Experimental Researches in Electricity (1839-1855), in which Faraday explained magnetism by force lines traversing space, instead of by actions at a distance. Thomson had cast some of Faraday's ideas into mathematical form and guided by his work Maxwell went a step further. In his first paper on electromagnetism (1855), entitled On Faraday's Lines of Force,[21] one of his aims was to overturn the idea of action at a distance.[22]
Maxwell presented a — what he called — geometrical model of lines of force in space. This representation rested on equipotential surfaces (scalar functions) to which the force lines (gradient vectors) are orthogonal and give paths of steepest descent from one equipotential surface to the next. Maxwell was guided by the physical analogy of incompressible fluids; not that Maxwell believed electricity to be a fluid, but he recognized the mathematical analogy of field lines to the velocity vectors of flowing fluids.
On Physical Lines of Force
Five years later, in 1861, Maxwell returned to the subject and wrote a series of four papers entitled On Physical Lines of Force.[23] In this work he introduced a mechanical ether model, based on "molecular vortices" and "idle wheel particles", that is rather foreign to the modern reader. However, he inferred two momentous consequences: the usual conduction current has to be augmented with a displacement current and the ether sustains transversal vibrations propagating with a velocity c, now known as the speed of light.
To put the second consequence into perspective it must be recalled that in 1856 c had been measured by Wilhelm Eduard Weber and Rudolf Kohlrausch as the factor connecting two different definitions of electric charge (see statcoulomb and abcoulomb): by Coulomb's law and by Ampère's equation. Almost immediately Gustav Kirchhoff noticed that their value of c was surprisingly close to the speed of light determined by Hippolyte Louis Fizeau in 1849. And now Maxwell had shown that electromagnetic waves propagate with exactly the same speed.
In the introduction of part III of his On Physical Lines of Force Maxwell puts forward the supposition that there is only one medium, one ether, carrying both light and electromagnetic phenomena. On p. 22 of the same paper, he writes the following paragraph which is one of the landmarks in the history of the exact sciences:
The velocity of transverse undulations in our hypothetical medium, calculated from the electro-magnetic experiments of MM. Kohlrausch and Weber, agrees so exactly with the velocity of light calculated from the optical experiments of M. Fizeau, that we can scarcely avoid the inference that light consists in the transverse undulations of the same medium which is the cause of electric and magnetic phenomena.
A Dynamical Theory of the Electromagnetic Field
In 1865 Maxwell wrote his most important paper "A Dynamical Theory of the Electromagnetic Field,[24] in which he introduces a set of equations, now known as the Maxwell equations. This paper is very accessible, also by the modern reader, because he abandoned his mechanical model of vortices and idle wheels, and only applies concepts that are still being taught in modern university courses. He presents twenty equations, divided in eight groups labeled (A) to (H), with twenty variables. Since he did not yet know vector or quaternion notation the different components of his vector fields are counted as individual parameters and the equations are written out in components. For a representation of his equations in modern vector form and comments on them, see the Addendum.
The Treatise
Maxwell's electrical work was ultimately summed up in his Treatise on Electricity and Magnetism[3]. The first edition appeared in 1873, the second in 1881, after the author's death, and was edited by W. D. Niven, although it had been revised in part by Maxwell. The third edition, 1891, was edited by Joseph John Thomson. The book is not a systematic treatise in the ordinary sense, and it would be hard to go through it from cover to cover. It contains lots of interesting material and insights, but this has to be dug out the hard way. Maxwell's equations appear only in Chapter IX of part 4, well into the second half of the second volume. Maxwell uses both component and quaternion notation. The book appeared to contemporary physicists, and even to the immediate following generations, as a great but inaccessible monument.
References and notes
- ↑ For instance, James's great-grandmother from father's side was Dorothea, Lady Clerk Maxwell. She was the daughter of William and Agnes (née Maxwell) Clerk Maxwell. Dorothea married Sir George Clerk and — as usual in those days — carried the married name Clerk, instead of her father's name Clerk Maxwell.
- ↑ Lewis Campbell and William Garnett (1882) The Life of James Clerk Maxwell MacMillan, London Online
- ↑ 3.0 3.1 J. Clerk Maxwell (1873) A Treatise on Electricity and Magnetism. Clarendon Press, Oxford.
- ↑ Tait PG (1985) Elementary Treatise on Quaternions Oxford
- ↑ Crowe MJ A History of Vector Analysis Dover, New York p. 129
- ↑ Lewis Campbell and William Garnett (1882) The Life of James Clerk Maxwell, MacMillan, London
- ↑ It is interesingt to note in this context that the competing colour theory (Zur Farbenlehre) of Young's famous German contemporary, Goethe, has had no impact on scientific colour theory.
- ↑ J. Clerk Maxwell(1861) On the theory of three primary colours Proc Royal Institution Great Britain 3:370-4
- ↑ Evans RM (1961) Maxwell’s colour photograph Scientific American 205:118–27
- ↑ Dougal RC et al. (2006) Then and now: James Clerk Maxwell and colour Optics Laser Technol38:210–8. An in-depth review of Maxwell's work on colour theory with a link to present day applications of colour science. http://dx.doi.org/10.1016/j.optlastec.2005.06.036
- ↑ Maxwell JC (1859) On the stability of the motion of Saturn's rings Monthly Notices, Astronomical Soc London 19:297-304
- ↑ Newman JR The World of Mathematics, vol. II, p. 774, excerpt from Daniel Bernoulli's book Hydrodynamica (1738)
- ↑ Clausius R (1850) On the mean length of the paths described by the separate molecules of gaseous bodies on the occurrence of molecular motion Phil Magazine, Ser. 4 17:81-91 (1859)
- ↑ Maxwell JC (1860) Illustrations of the dynamical theory of gases I. On the motions and collisons of perfectly elastic spheres Phil. Magazine, Ser. 4 19:19-32; II. On the process of diffusion of two or more kinds of moving particles among one another Phil. Magazine, Ser. 4 20:21-37
- ↑ J. Clerk Maxwell, On the dynamical Theory of Gases, read May 31, 1866; published in Philosophical Transactions of the Royal Society of London, vol. 157, pp. 49-88 (1867)
- ↑ J. Clerk Maxwell, Theory of Heat, Longmans, Green, London (1871); reprinted Dover, New York (2001). Maxwell wrote about a "finite being", the name "demon" was coined by W. Thomson in 1874.
- ↑ One can confirm this easily for a monoatomic ideal gas by direct calculation. The energy of one mole of such gas is 3RT/2, with R the molar gas constant and T the absolute temperature. The entropy of one mole is S(T) = R ln(V•T 3/2) + S(0). Assume that the amount of gas in A and B stays constant (one mole each) during the demon's operation and that both partitions have the same volume V. By conservation of energy
- ↑ L. Szilard, Über die Entropieverminderung in einem thermodynamischen System bei Eingriffen intelligenter Wesen. [On the decrease of entropy in a thermodynamic system by the intervention of intelligent beings]. Zeitschrift für Physik, vol. 53, pp. 840-856 (1929) doi
- ↑ Maxwell's Demon 2, Entropy, Classical and Quantum Information, Computing edited by Harvey S. Leff and Andrew F. Rex, Institute of Physics Publishing, Bristol, 2nd edition, 2003
- ↑ Maxwell's demon - blog post on the history of thermodynamics, with detailed treatment of the demon
- ↑ J. Clerk Maxwell, On Faraday's Lines of Force, Trans. Cambridge Philosophical Society, vol. 10, pp. 27-83 (1855)
- ↑ P. G. Tait in a posthumous article on Maxwell in the 1911 edition of the Encyclopaedia Britannica. Online
- ↑ J. Clerk Maxwell, On Physical Lines of Force, Philosophical Magazine 4th series, Four Parts, I: vol. 11, pp. 161-175; II: vol. 11, pp. 281-291, 338-347; III: vol. 13, pp. 12-23; IV: vol. 13, pp. 85-95 (1861, 1862).online
- ↑ J. Clerk Maxwell, A Dynamical Theory of the Electromagnetic Field" Phil. Trans. Roy. Soc 155:459 - 512 (1865) online)






![{\displaystyle \scriptstyle \Delta S^{A}+\Delta S^{B}={\frac {3}{2}}\,R\,\ln {\Big [}1-\left({\frac {\Delta T^{A}}{T}}\right)^{2}{\Big ]}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/20dda71b052df599fc58e053d8d8510194805b0a)