Law of cosines: Difference between revisions
Jump to navigation
Jump to search
imported>David E. Volk No edit summary |
imported>David E. Volk (need help, image won't show up) |
||
| Line 2: | Line 2: | ||
<math> c^2 = \left(a^2 + b^2\right) - \left(2ab\right)cos(C) </math> | <math> c^2 = \left(a^2 + b^2\right) - \left(2ab\right)cos(C) </math> | ||
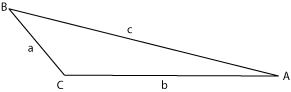
where a, b and c are the sides of the triangle opposite to angles A, B and C, respectively (see image). | |||
[[Image:Triangle.jpg|center|frame|Triangle]] | [[Image:Triangle.jpg|center|frame|Triangle]] | ||
Revision as of 16:31, 3 October 2007
The law of cosines is a useful identity for determining an angle or the length of one side of a triangle, when given two angles and three lenghts or three angles and two lengths, respectively. When dealing with a right triangle, the law of cosines reduces to the Pythagorean theorem because the cos(90) is zero. To determine the areas of triangles, see the Law of sines.
where a, b and c are the sides of the triangle opposite to angles A, B and C, respectively (see image).