Sine rule: Difference between revisions
imported>Paul Wormer (→Lemma) |
imported>Paul Wormer No edit summary |
||
| Line 3: | Line 3: | ||
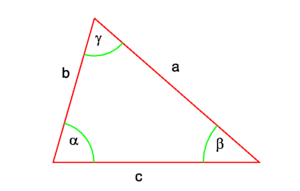
In [[trigonometry]], the '''sine rule''' (also known as '''[[Law of sines|Law of Sines]]''') states that the ratio of the sines of the angles of a triangle is equal to the ratio of the lengths of the opposite sides, see Fig.1. Equivalently, | In [[trigonometry]], the '''sine rule''' (also known as '''[[Law of sines|Law of Sines]]''') states that the ratio of the sines of the angles of a triangle is equal to the ratio of the lengths of the opposite sides, see Fig.1. Equivalently, | ||
:<math> | :<math> | ||
\frac{a}{\sin\alpha} = \frac{b}{\sin\beta}= \frac{c}{\sin{\gamma}} | \frac{a}{\sin\alpha} = \frac{b}{\sin\beta}= \frac{c}{\sin{\gamma}} = d, | ||
</math> | </math> | ||
where ''d'' is the diameter of the circle circumscribing the triangle. | |||
==Proof== | ==Proof== | ||
The easiest proof is purely geometric. | The easiest proof is purely geometric. | ||
Revision as of 09:31, 18 October 2008
In trigonometry, the sine rule (also known as Law of Sines) states that the ratio of the sines of the angles of a triangle is equal to the ratio of the lengths of the opposite sides, see Fig.1. Equivalently,
where d is the diameter of the circle circumscribing the triangle.
Proof
The easiest proof is purely geometric.
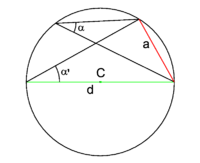
Lemma
In Fig. 2 the angle β satisfies,
This follows because the two angles, α and β, in Fig. 2 share a segment of the circle (have the chord a in common). By a well-known theorem of plane geometry it follows that the two angles are equal. The angle α, having the diameter of the circle d as one of its sides, has as opposite angle a right angle. Hence sin(α) = a/d, the length of chord a divided by the diameter d. It follows that the angle β, with a corner on the circumference of the same circle as α, but other than that arbitrary, has the same sine as α.
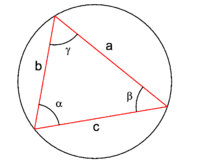
Proof of sine rule
From the lemma follows that the angles in Fig. 3 are
where d is the diameter of the circle. From this result the sine rule follows.