Law of sines
In trigonometry, the law of sines (also known as sine rule) relates in a triangle the sines of the three angles and the lengths of their opposite sides,
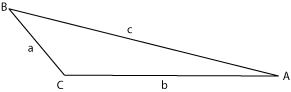
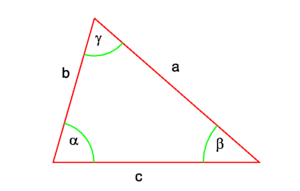
where d is the diameter of the circle circumscribing the triangle and the angles and the lengths of the sides are defined in Fig. 1 for an obtuse-angled triangle and in Fig. 2 for an acute-angled triangle. From the law of sines follows that the ratio of the sines of the angles of a triangle is equal to the ratio of the lengths of the opposite sides.
The rule is useful to determine unknown angles and sides of a triangle when any of the following three elements is given:
- One side, the opposite angle, and one adjacent angle.
- One side and two adjacent angles
- Two sides and an angle not included by the sides.
The rule
may be useful in such a determination.
The use of the law of sines is complementary to the use of the law of cosines.
Proof
The easiest proof is purely geometric, not algebraic.
Lemma
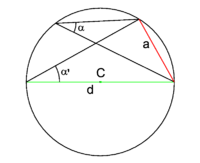
In Fig. 3 the arbitrary angle α satisfies,
where d is the diameter of the circle and a is the chord opposite α. To prove this we consider the angle α' that has the diameter of the circle as one of its sides, see Fig. 3. The two angles, α and α' share a segment of the circle (have the chord a in common). The angle α', having the diameter of the circle d as one of its sides, has as opposite angle a right angle. Hence sin(α') = a/d, the length of chord a divided by the diameter d. A well-known theorem of plane geometry states that α = α' and it follows that the angle α has the same sine as α'.
Proof of sine rule
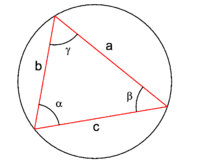
From the lemma follows that the angles in Fig. 4 are
where d is the diameter of the circle. This proves the sine rule.