Tetration

Fig.1. Tetration $\mathrm {tet} _{b}(x)$ for $b=\mathrm {e}$, $b=2$, $b=\exp(1/\mathrm {e} )$, and $b={\sqrt {2}}$ versus $x$.

This article is currently under construction. While, use article from wikipedia http://en.wikipedia.org/wiki/Tetration

Tetration is fastly growing mathematical function, which was introduced in XX century and suggested for representation of huge numbers in mathematics of computation. However, up to year 2008, this function is not considered as elementary function, it is not implemented in programming languages and it is not used for the internal representation of data, at least in the commercial software.

Definiton

For real $b>1$, Tetration $F=\mathrm {tet} _{b}$ on the base $b$ is function of complex variable, which is holomorphic at least in the range $\{z\in \mathbb {C} :~\Re (z)>-2\}$, bounded in the range $\{z\in \mathbb {C} :~|\Re (z)|\leq 1\}$, and satisfies conditions

$F(z+1)=\exp _{b}\!{\big (}F(z){\big )}$

$F(0)=1$

$F\!{\big (}z^{*}{\big )}=F{\big (}z{\big )}^{*}$

at least within range $\Re (z)>-2$.

Etymology

Creation of word tetration is attributed to Englidh mathematician Reuben Louis Goodstein . This name indicates, that this operation is fourth (id est, tetra) in the hierarchy of operations after summation, multiplication, and exponentiation. In principle, one can define "pentation", "sexation", "septation" in the simlar manner, although tetration, perhaps, already has growth fast enough for the requests of XXI century.

Real values of the arguments

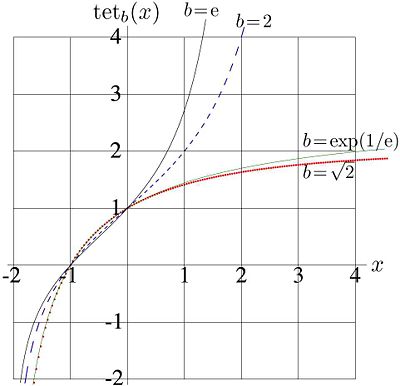
Examples of behavior of this function at the real axis are shown in figure 1 for values $b=\mathrm {e}$, $b=2$, $b=\exp(1/\mathrm {e} )$, and for $b={\sqrt {2}}$. It has logarithmic singularity at $-2$, and it is monotonously increasing function.

At $b\leq \exp(1/\mathrm {e} )$ tetration $\mathrm {tet} _{b}(x)$ approaches its limiting value as $x\rightarrow +\infty$, and $\displaystyle \lim _{x\rightarrow +\infty }~\mathrm {tet} _{b}(x)>1$.

At $b>\exp(1/\mathrm {e} )$ tetration $\mathrm {tet} _{b}(x)$ grows faster than any exponential function. For this reason the tetration is suggested for the representation of huge numbers in mathematics of computation. A number, that cannot be stored as floating point, could be stored as $\mathrm {tet} _{b}(x)$ for some standard value of $b$ (for example, $b=2$ or $b=\mathrm {e}$) and relatively moderate value of $x$. The analytic properties of tetration could be used for the implementation of arithmetic operations with huge numbers without to convert them to the floating point representation.

Integer values of the argument

For integer $z$, tetration ${\rm {tet}}_{b}$ can be interpreted as iterated exponential:

$\mathrm {tet} _{b}(1)=b$

$\mathrm {tet} _{b}(2)=b^{b}$

$\mathrm {tet} _{b}(3)=b^{b^{b}}$

and so on; then, the argument of tetration can be interpreted as number of exponentiations of unity. From definition it follows, that

$\mathrm {tet} _{b}(0)=1$

and

$\mathrm {tet} _{b}(-\!1)=0$

Relation with Ackermann funcitons

At base $b=2$, tetration is related to the Ackermann function:

$\mathrm {tet} _{2}(n+3)=A(4,n)+3$

where Ackermann function is defined for the positive integer values of its arguemtnrs with equations

$A(m,n)=\left\{{\begin{array}{llll}n+1&,&{\rm {if~}}~m\!=\!0\\A{\big (}m\!-\!1,~1~{\big )}&,&{\rm {if~}}m\!>\!0{\mbox{ and }}n\!=\!0\\A{\Big (}m\!-\!1,A(m,n\!-\!1){\Big )}&,&{\rm {if~}}m\!>\!0{\mbox{ and }}n\!>\!0\end{array}}\right.$

Evaluation of tetration

As the asymptoric of tetration is crutually depend of base $b$ in ficinity of value $b=\exp(1/\mathrm {e} )$, the evaluation procidure is different foe the cases $1<b<\exp(1/\mathrm {e} )$, $b=\exp(1/\mathrm {e} )$, and $b>\exp(1/\mathrm {e} )$, and should be considered intependently.

Existence and uniqueness of tetration

This section is not yet written. There is non-finished draft at User:Dmitrii Kouznetsov/Analytic Tetration.

Iterated exponential and ${\sqrt {\exp }}$

Especially interesting, and in particular, for the qualitative breakthrough, id the case of iteration of natural exponential, id est, $b=\mathrm {e} )$. Existence of the fractional iteration, and, in particular, existence of operation ${\sqrt {\exp }}=\exp ^{1/2}$ was demonstrated in 1950 by H.Kneser. . However, that time, there was no computer facility for the evlauation of such an exotic function $F$ that $F(F(z))=\exp(z)$; perhaps, just absense of an apropriate plotter did not allow Kneser to plot the distribution of fractal exponential function $\exp ^{c}(z)$ in the complex $z$ plane for various values of $c$.

Free online sources

• http://reglos.de/lars/ffx.html , Lars Kindermann. References about Iterative Roots and Fractional Iteration.
• http://math.eretrandre.org/tetrationforum/index.php , Discussion about tetration
• http://tetration.itgo.com/ Tetration site by Andrew Robbins
• http://www.tetration.org/ Tetration site by Daniel Geisler