Faraday's law (electromagnetism)

In electromagnetism Faraday's law of magnetic induction states that a change in magnetic flux generates an electromotive force. The law is named after the English scientist Michael Faraday.

CC Image
Magnetic flux. The flux through surface S₁ (yellow) is equal to the flux through surface S₂ (gray). Both surfaces have the contour C (red) as boundary.

The magnetic flux Φ through a surface S is defined as the surface integral

$\Phi \equiv \iint _{S}\mathbf {B} \cdot d\mathbf {S} ,$

where dS is a vector normal to the infinitesimal surface element dS and dS is of length dS. The dot stands for the inner product between the magnetic induction B and dS. In vacuum the magnetic induction B is proportional to the magnetic field H. (In SI units: B = μ₀ H with μ₀ the magnetic constant of the vacuum; in Gaussian units: B = H.)

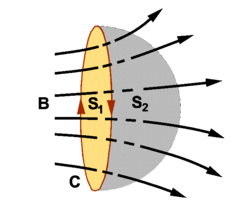
The flux through two surfaces that together form a closed surface is equal because of Gauss' law. Indeed, in the figure on the right the surfaces S₁ and S₂, which have the boundary C in common, form together a closed surface. Hence Gauss' law states that

$0=-\iint _{S_{1}}\mathbf {B} \cdot d\mathbf {S} +\iint _{S_{2}}\mathbf {B} \cdot d\mathbf {S} ,$

where the minus sign of the first term is due to the fact that the flux is into the volume enveloped by the two surfaces. It follows that

$\Phi =\iint _{S_{1}}\mathbf {B} \cdot d\mathbf {S} =\iint _{S_{2}}\mathbf {B} \cdot d\mathbf {S} ,$

and that the magnetic flux Φ can be computed with respect to any surface that has C as boundary.

The electromotive force (EMF) is defined as

${\mathcal {E}}\equiv \oint _{C}\mathbf {E} \cdot d\mathbf {l} ,$

where the electric field E is integrated around the closed path C.

Faraday's law of magnetic induction relates the EMF $\scriptstyle {\mathcal {E}}$ to the time derivative of the magnetic flux, it reads:

${\mathcal {E}}=-k{\frac {d\Phi }{dt}}\quad {\textrm {with}}\quad k={\begin{cases}1\quad &{\hbox{SI units}}\\1/c&{\hbox{Gaussian units}},\end{cases}}$

where c is the speed of light. If C is a conducting loop, then under influence of the EMF a current i_ind will run through it. The minus sign in Faraday's law has the consequence that the magnetic field generated by i_ind opposes the change in Φ this phenomenon is known as Lenz' law. If the surface S is constant the change in Φ is solely due to a change in B.

Faraday's law forms the theoretical basis of the electric motor, the dynamo, and the electric generator.

Connection to Lorentz force

CC Image
Conducting bar moves to the right on conducting rails in homogeneous field B. Surface grows with v. Lorentz force is downward and gives an electric current i_ind in clockwise direction around the closed circuit.

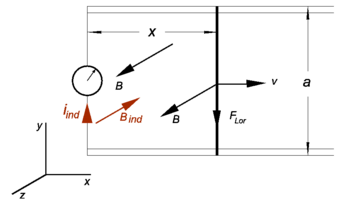
It is instructive to derive Faraday's law from the Lorentz force for a special case. To that end an experimental setup is depicted in the figure on the right. A conducting bar of length a moves in positive x direction over two parallel pairs of conducting rails. The speed v of the bar is constant. The whole setup is placed in a homogeneous magnetic field B = μ₀ H (we are using SI units) that points toward the reader, i.e., in positive z-direction. Homogeneity means that everywhere in the plane of drawing a magnetic field of the same strength points toward the reader.

The magnetic field is constant, but the surface S increases linearly in time

$S=xa\Longrightarrow {\frac {dS}{dt}}={\frac {dx}{dt}}a\equiv va.$

The vector B points in positive z-direction and so does the normal to S,

${\begin{aligned}{\frac {d}{dt}}\iint _{S}\mathbf {B} \cdot d\mathbf {S} &={\frac {d}{dt}}\iint _{S}BdS\\&=B{\frac {d}{dt}}\iint _{S}dS=B{\frac {dS}{dt}}=Bva.\end{aligned}}$

The time-dependence of the growing flux is solely due to the time-dependence of the growth of the surface. The time derivative of the magnetic flux in the figure is positive and equal to

${\frac {d\Phi }{dt}}=Bva.\qquad \qquad \qquad \qquad (1)$

The Lorentz force (in SI units) is the cross product,

$\mathbf {F} _{\mathrm {Lor} }=\mathbf {v} \times \mathbf {B} =-\mathbf {e} _{y}vB.$

This constant force acts over the length a of the moving bar and gives the electromotive force (EMF) equal to force times path length,

${\mathcal {E}}=-vBa\qquad \qquad \qquad \qquad (2).$

From equations (1) and (2) follows

${\mathcal {E}}=-{\frac {d\Phi }{dt}},$

which indeed is Faraday's law of magnetic induction.

The EMF gives a current i_ind in the direction of the Lorentz force, i.e., in a clockwise direction in the plane of drawing (the x-y plane). By Biot-Savart's law a current gives a circular magnetic field. The current i_ind has a tangent vector in the x-y plane that is in negative z-direction. Hence B_ind opposes B, in accordance with Lenz' law.