Displacement current

In physics, more particular in the theory of electromagnetism, the displacement current is the time derivative of the electric displacement D. It was introduced by James Clerk Maxwell as a correction to Ampère's law. It is the displacement current that gives rise to electromagnetic waves.

Explanation

Charging of a parallel-plate capacitor (green plates) with current i. V indicates a volume enveloped by a closed surface.

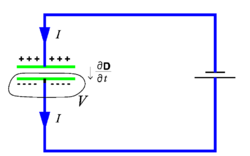
In the figure on the right a capacitor is charged by an electric current i. The conduction current i is related to the current density j(r) by a surface integral. Since j is everywhere zero except in the wire (blue in the figure), one may integrate over the whole closed surface of the volume V,

$i=\iint _{S}\mathbf {j} \cdot d\mathbf {S} =\iiint _{V}{\boldsymbol {\nabla }}\cdot \mathbf {j} \,dv,$

where the last equality follows by application of the divergence theorem of Gauss. The surface integral contains the inner product between the current density j (a vector) and a vector orthogonal to the infinitesimal surface element dS. The orthogonal vector has length dS. The conduction current i, which gives the electric charge leaving the volume V, is clearly non-zero during the time that the capacitor is charging.

Ampère's law in differential form states that

${\boldsymbol {\nabla }}\times \mathbf {H} =\mathbf {j} ,$

where H is the magnetic field. Since the divergence of the curl is zero,

${\boldsymbol {\nabla }}\cdot ({\boldsymbol {\nabla }}\times \mathbf {H} )={\boldsymbol {\nabla }}\cdot \mathbf {j} =0,$

it follows that

$i=\iiint _{V}{\boldsymbol {\nabla }}\cdot \mathbf {j} \,dv=0$

at all times, in contradiction to what was just stated. It follows that Ampère's law is in error, or is at least incomplete.

Maxwell was the first to notice this and he also saw how to fix it by application of the continuity equation. This equation states that the rate of change of charge density is proportional to the the divergence of the current density

${\boldsymbol {\nabla }}\cdot \mathbf {j} +{\frac {\partial \rho }{\partial t}}=0.$

The electrostatic law of Gauss states

${\boldsymbol {\nabla }}\cdot \mathbf {D} (\mathbf {r} )=\rho (\mathbf {r} ),$

so that

${\frac {\partial \rho }{\partial t}}={\boldsymbol {\nabla }}\cdot {\frac {\partial \mathbf {D} (\mathbf {r} )}{\partial t}},\qquad {\hbox{and}}\qquad {\boldsymbol {\nabla }}\cdot \left(\mathbf {j} +{\frac {\partial \mathbf {D} (\mathbf {r} )}{\partial t}}\right)=0.$

Maxwell saw that the conduction current leaving the volume V was compensated by the electric displacement current entering V, so he added the displacement current to the conduction current density, modifying Ampère's law to

${\boldsymbol {\nabla }}\times \mathbf {H} =\mathbf {j} +{\frac {\partial \mathbf {D} (\mathbf {r} )}{\partial t}},\qquad \qquad \qquad \qquad (1)$

with

${\boldsymbol {\nabla }}\cdot ({\boldsymbol {\nabla }}\times \mathbf {H} )={\boldsymbol {\nabla }}\cdot \left(\mathbf {j} +{\frac {\partial \mathbf {D} (\mathbf {r} )}{\partial t}}\right)=0.$

Equation (1) is one of Maxwell's equations formulated by him in 1864 and still accepted as universally valid.